「ヘリウム原子のエネルギー固有状態の計算方法」に基づいて、ヘリウム原子のエネルギー準位と固有関数の空間分布を計算したよ。「ヘリウム原子の基底状態の計算結果」で示したとおり、計算結果はよく知られた精密な実験結果とかなり一致しているよ。
次の図は、パラ（対称関数・スピン１重項）とオルト（反対称関数・スピン３重項）の主量子数 $n=1,2,3$ のエネルギー準位だよ。オルトのほうがパラよりも若干小さな値となるね。これは交換相互作用の結果だね。イオン化エネルギーは、電子２個の基底状態から電子１個を引き離すために必要なエネルギーで、「基底状態エネルギー（$-79.18[{\rm eV}]$）」から「電子が１個のみのヘリウム原子の基底状態エネルギー（ $-54.4[{\rm eV}]$ ）」で計算できるよ。
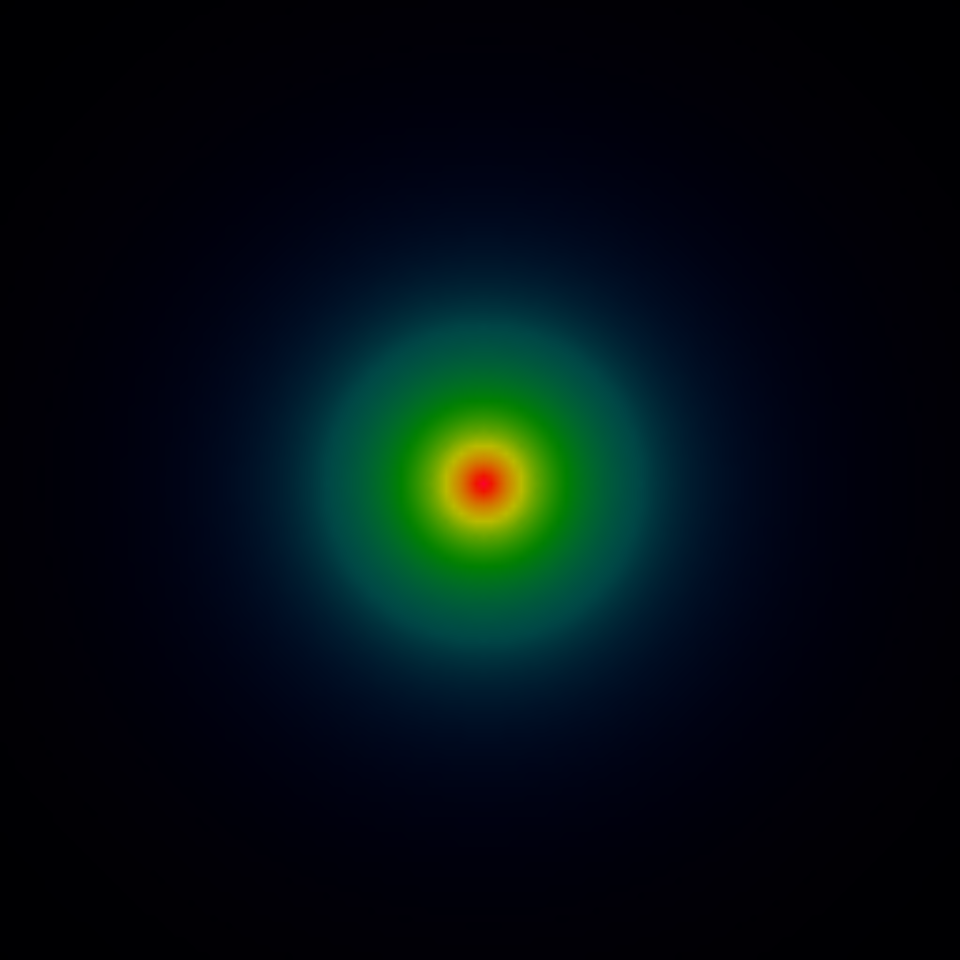
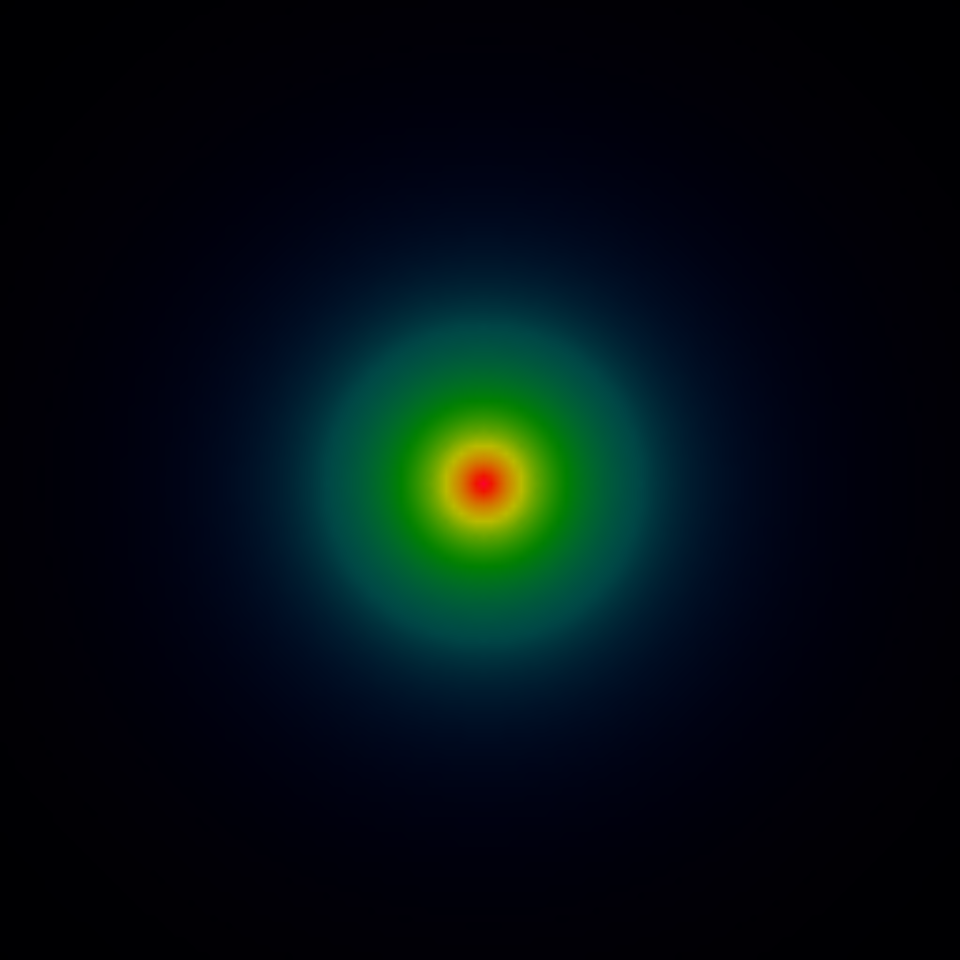
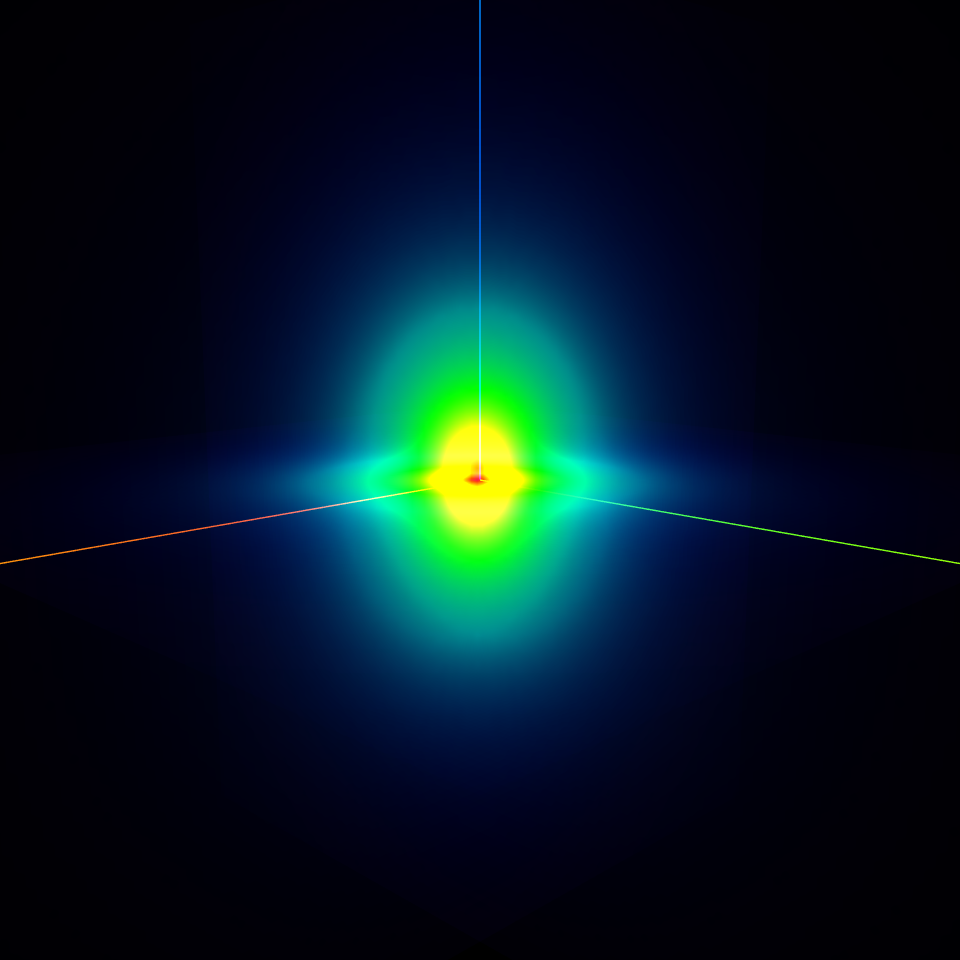
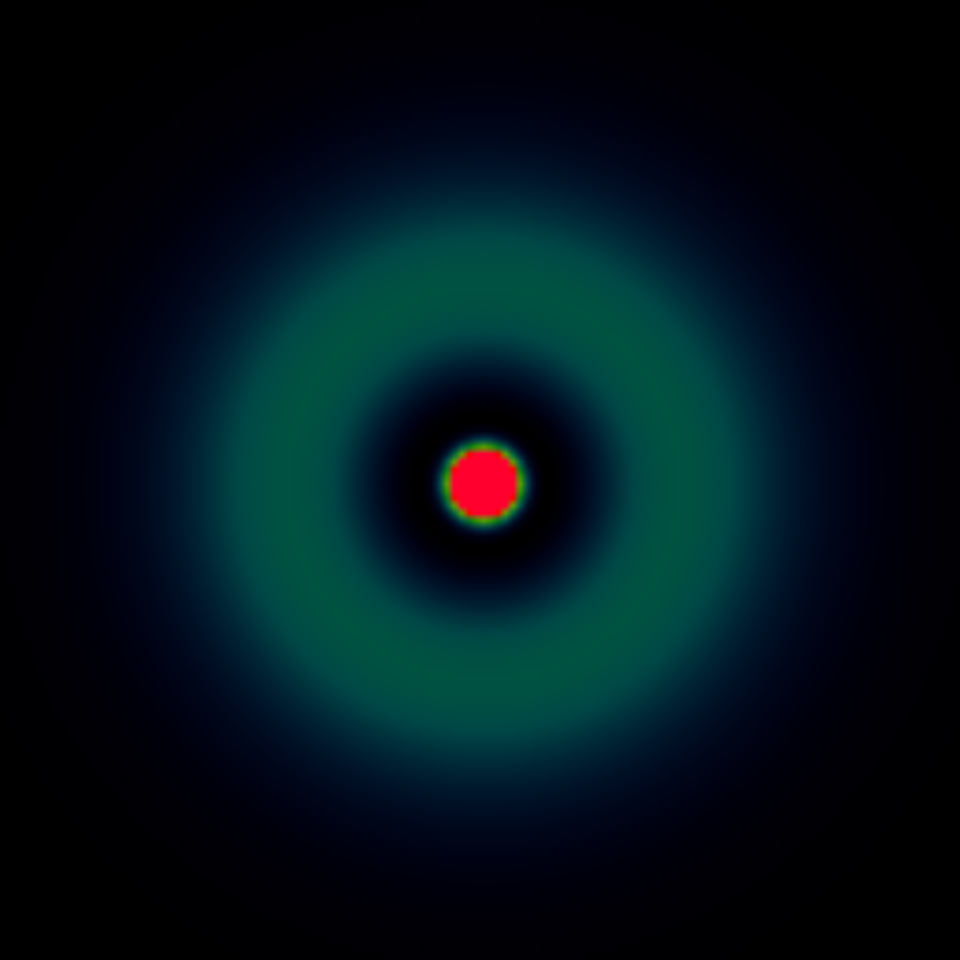
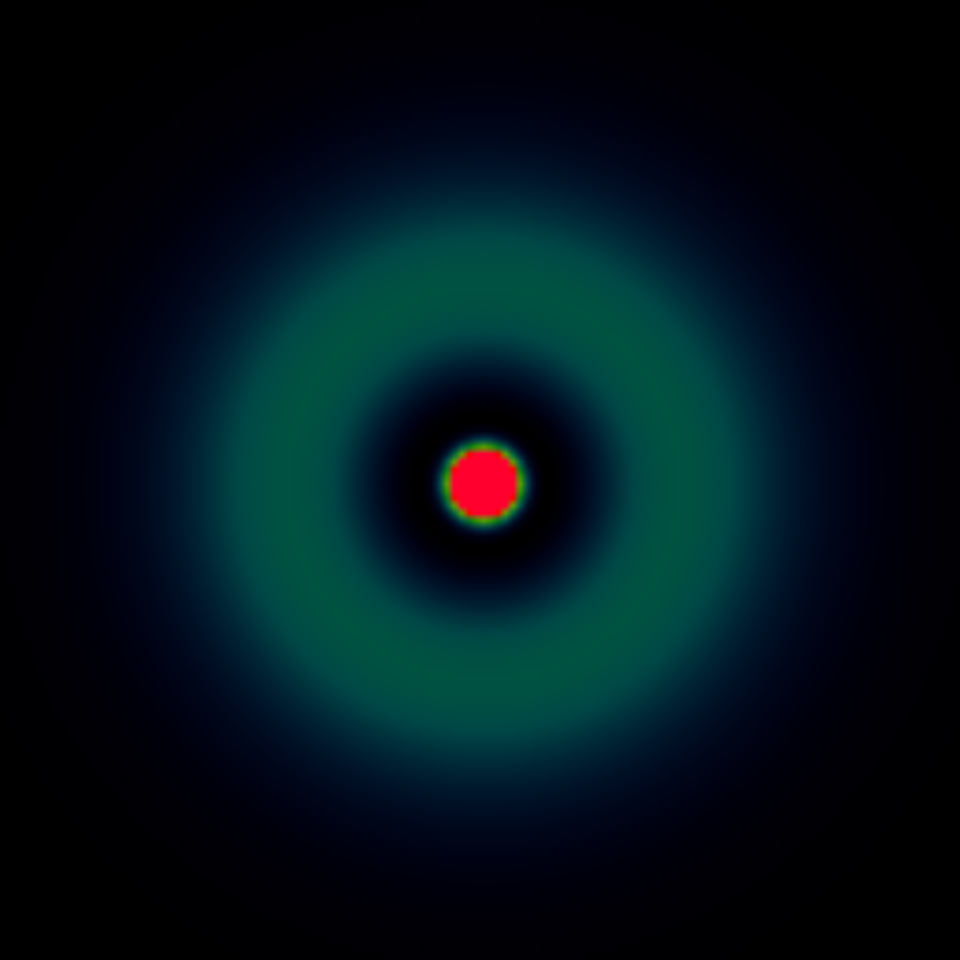
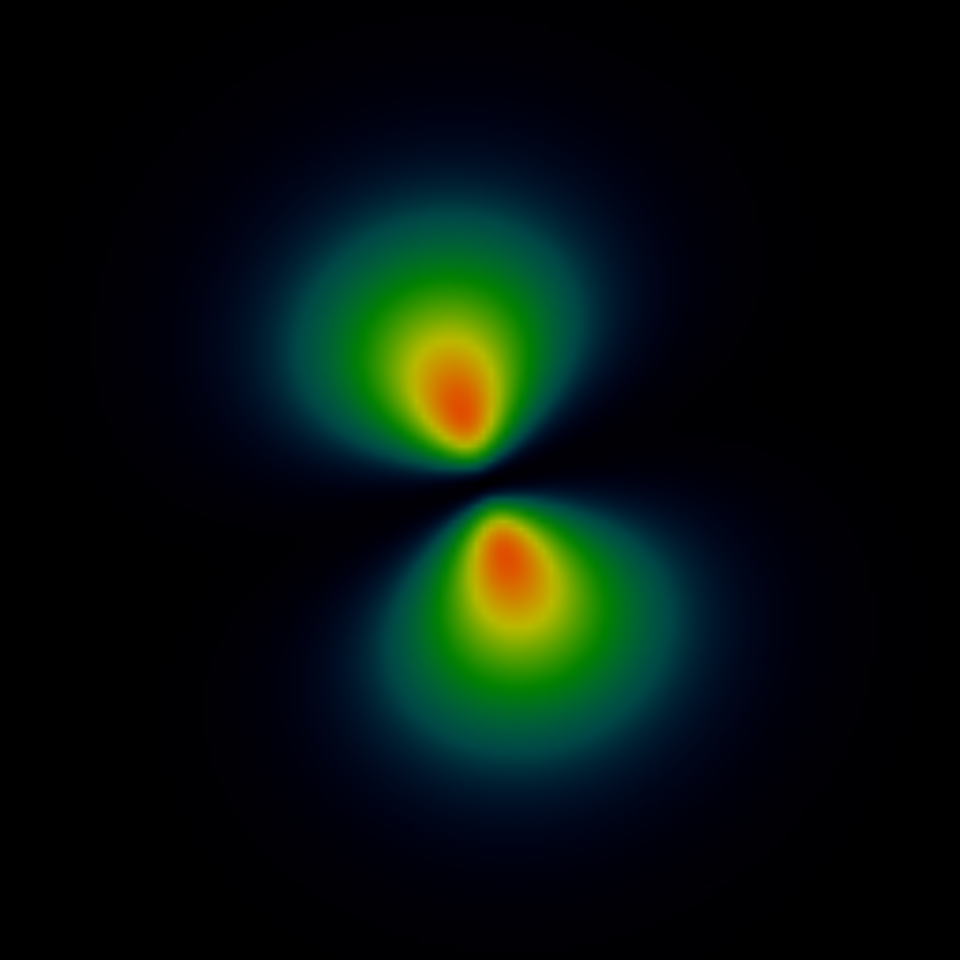
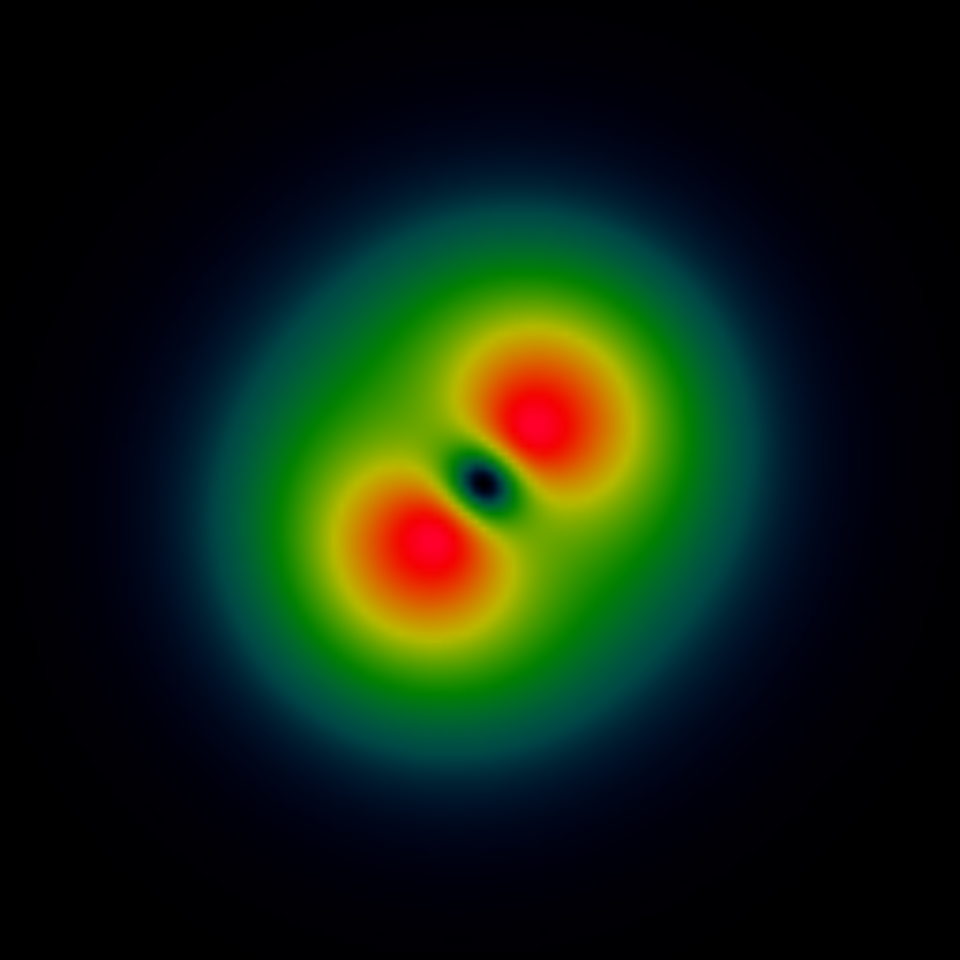
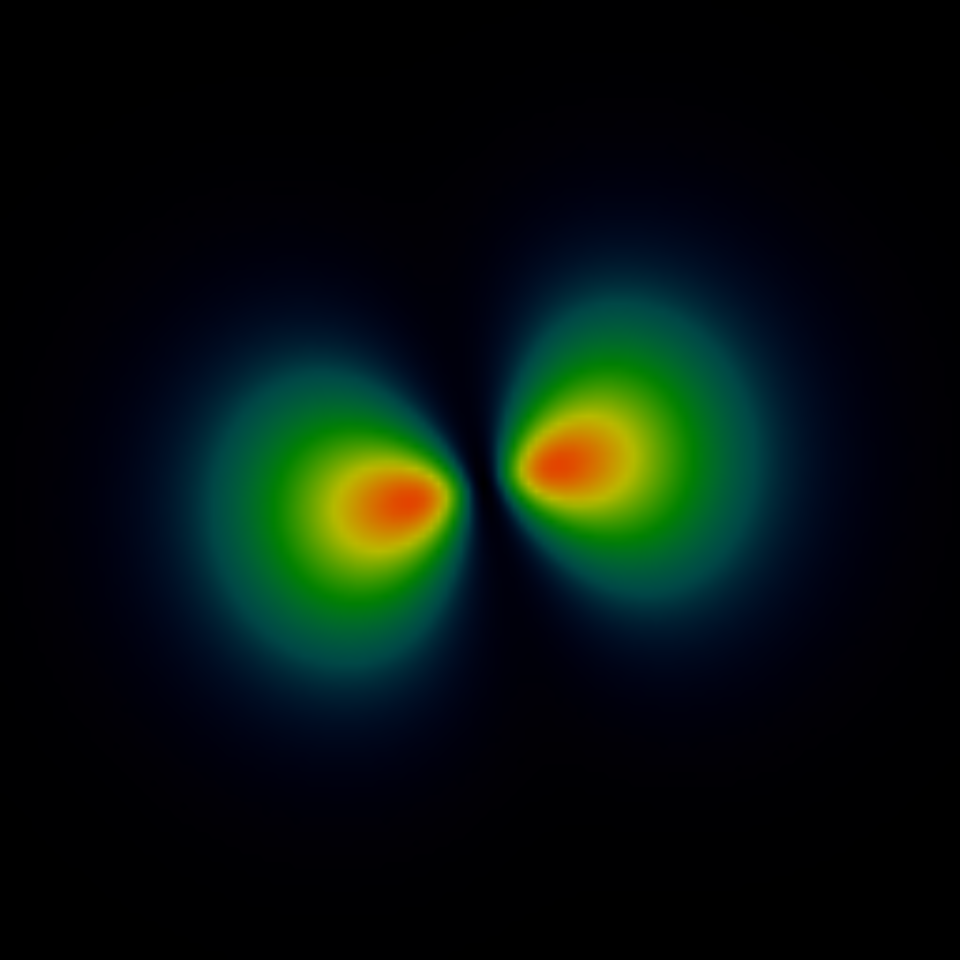
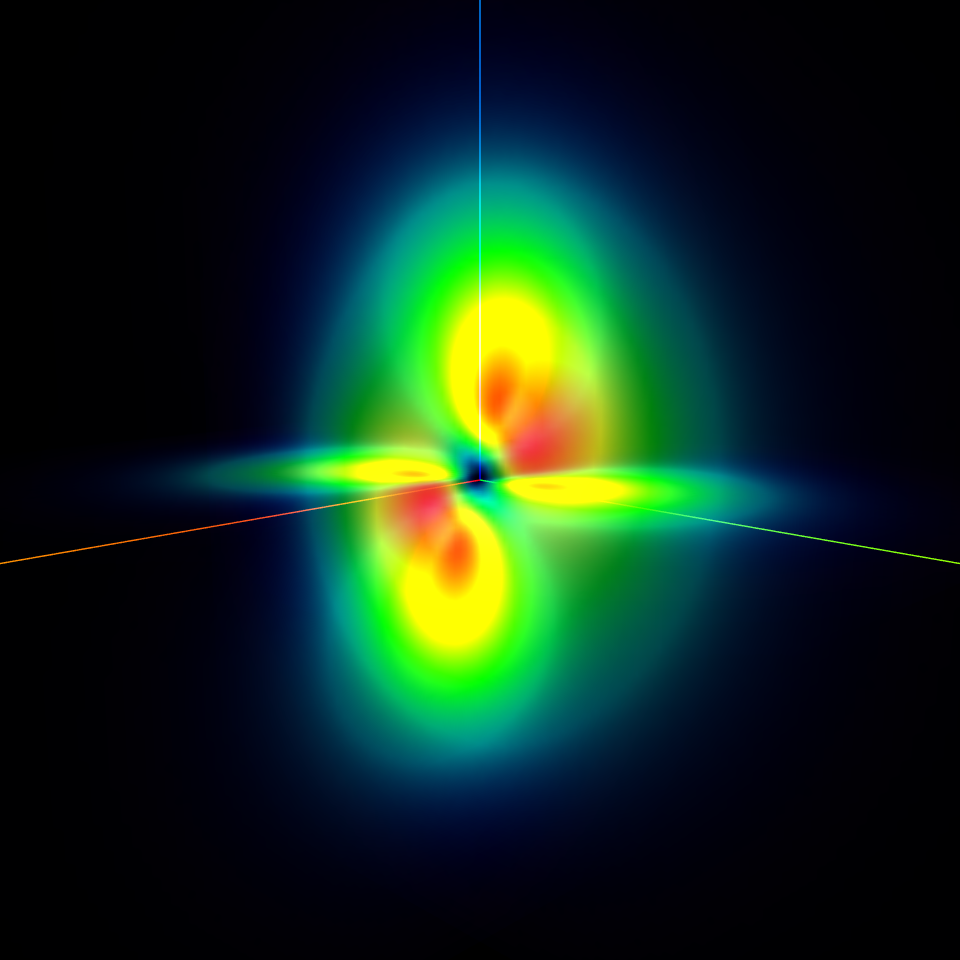
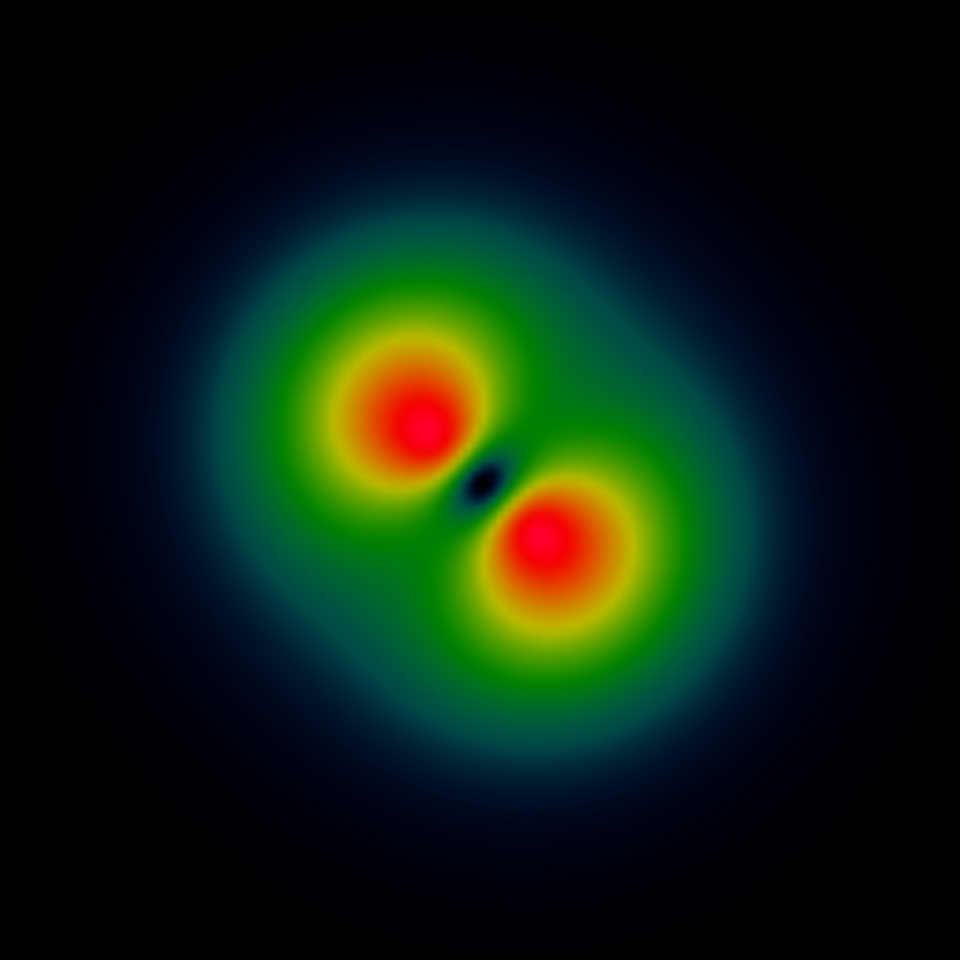
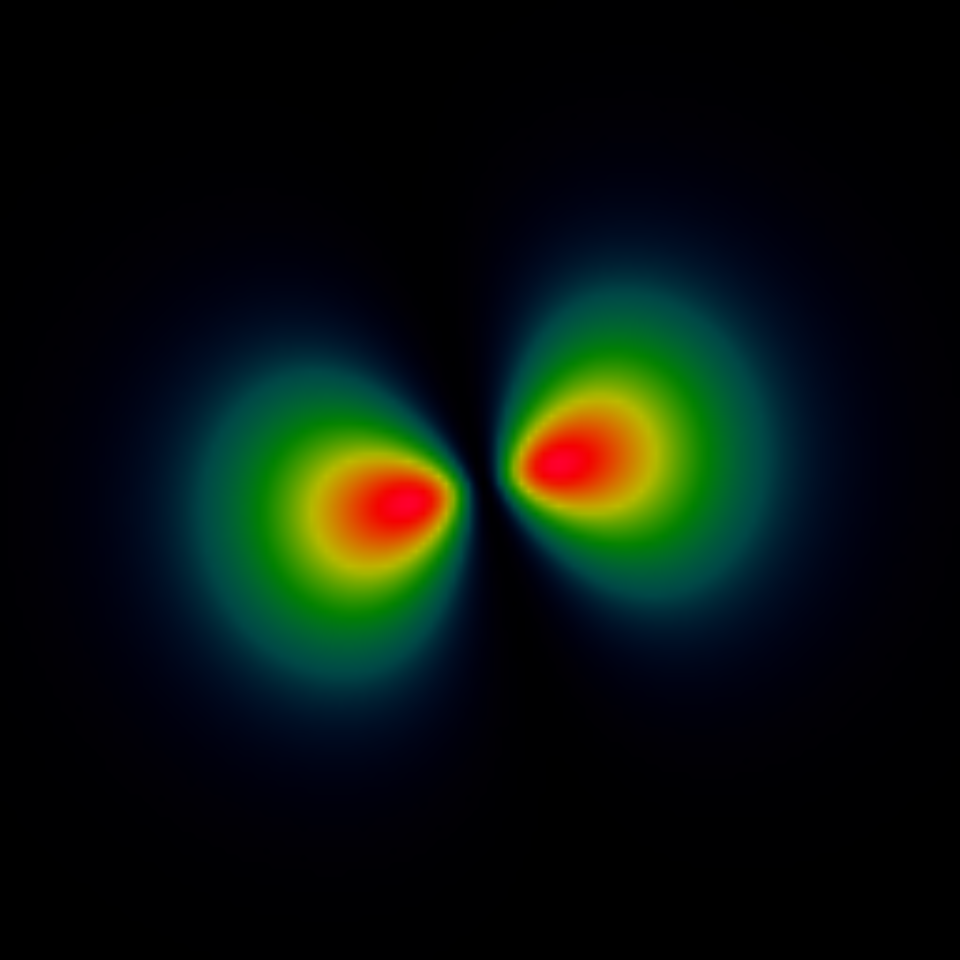
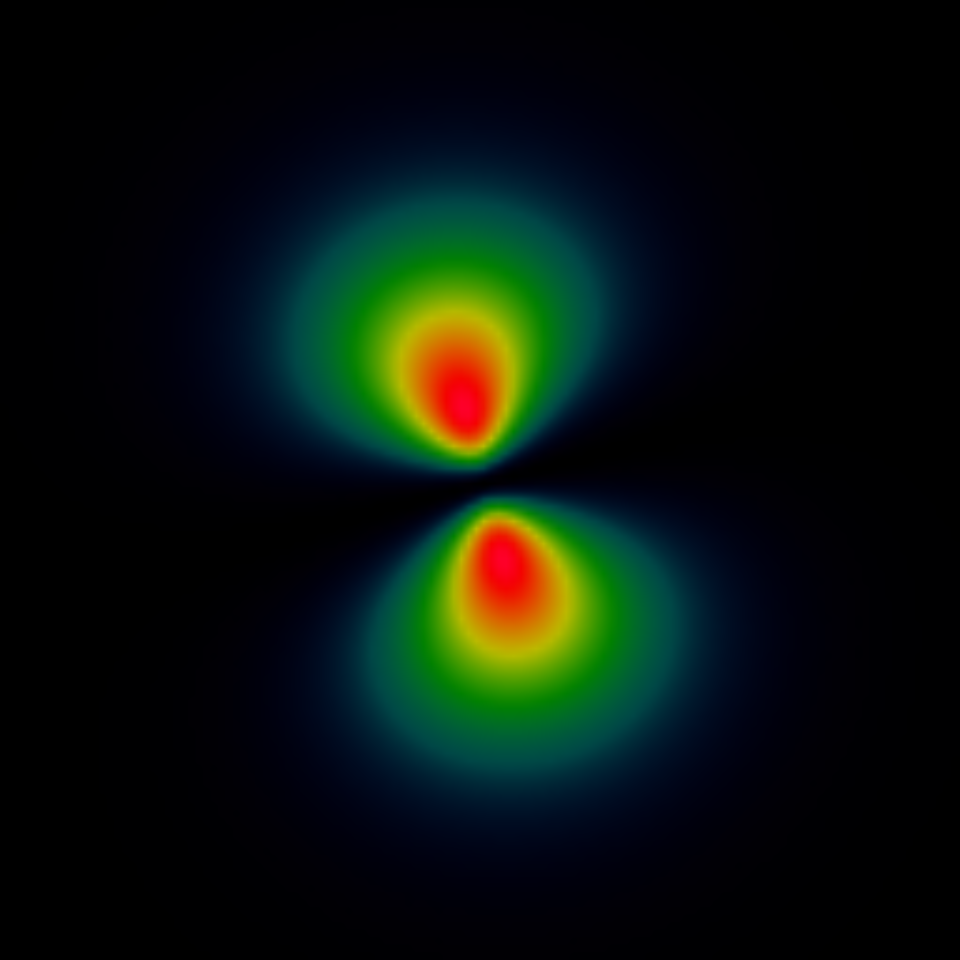
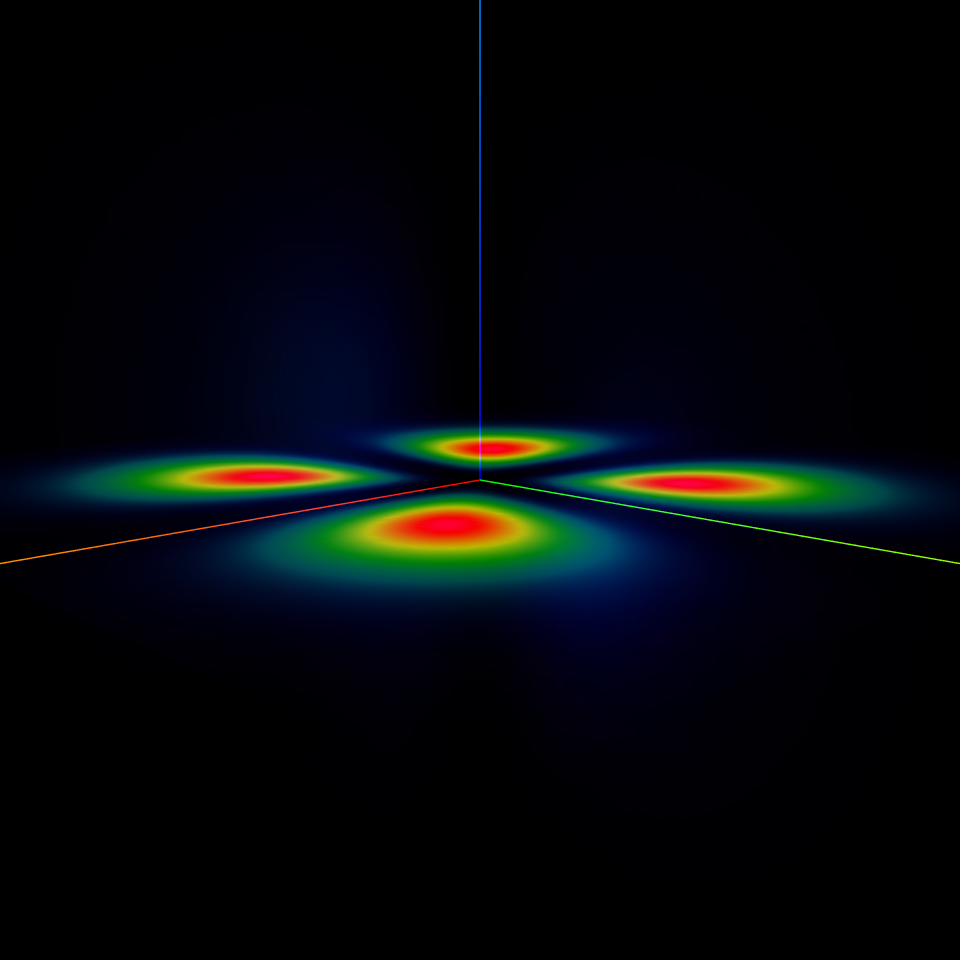
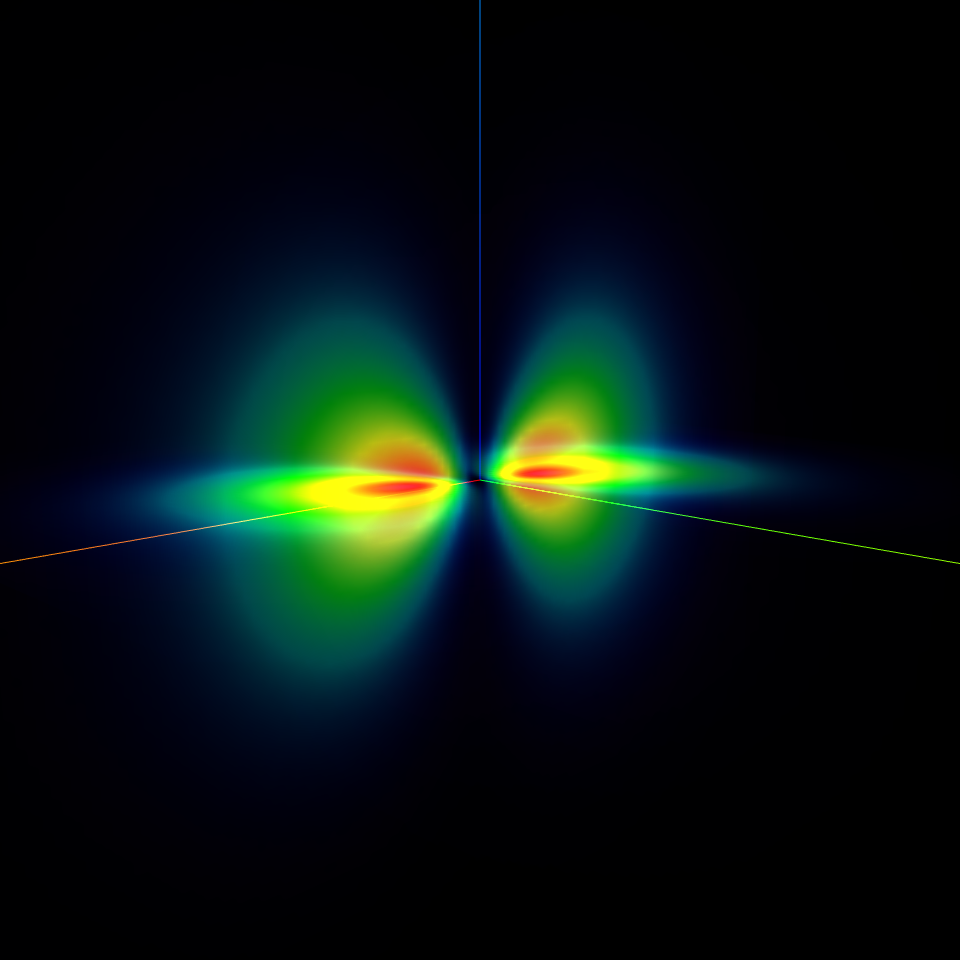
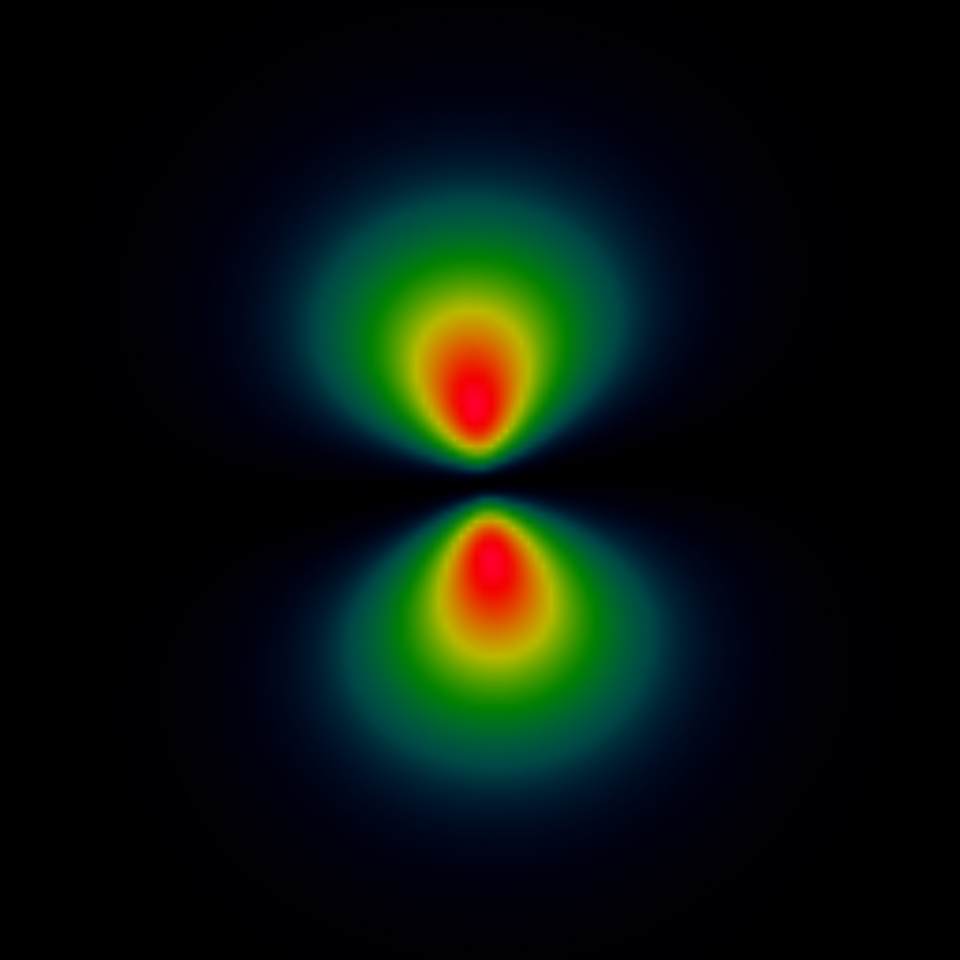
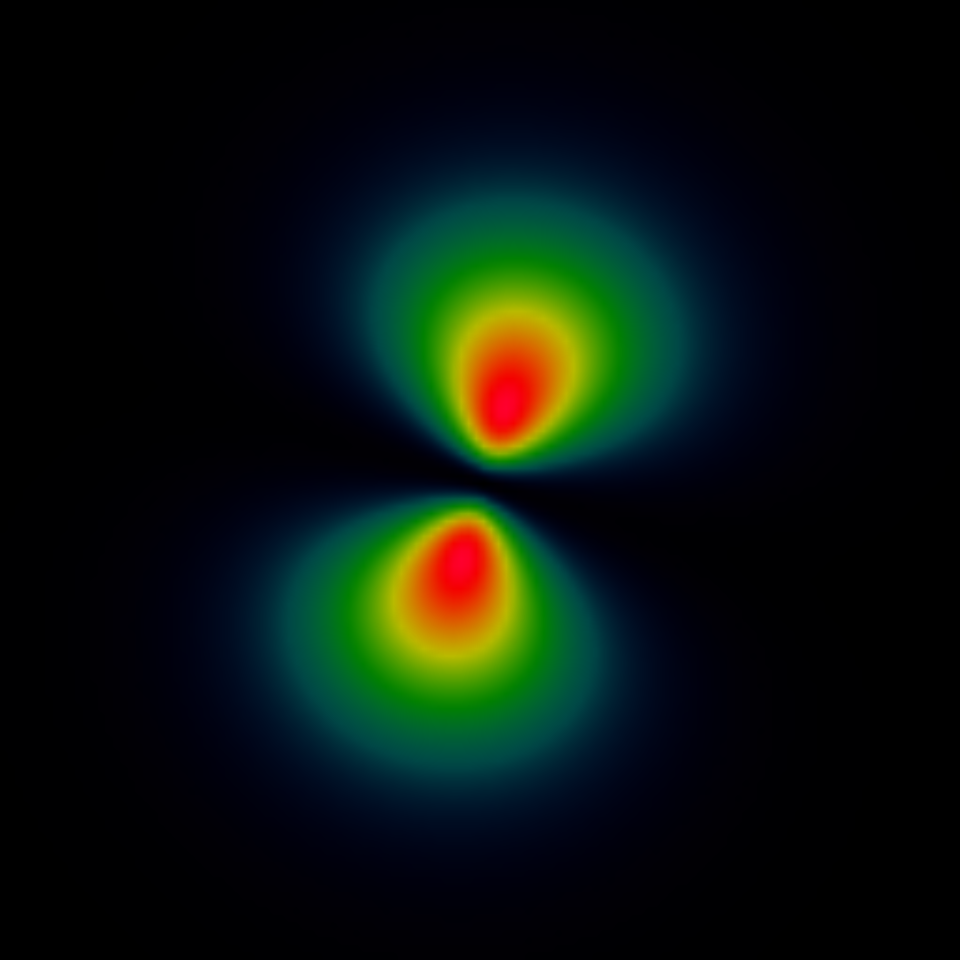
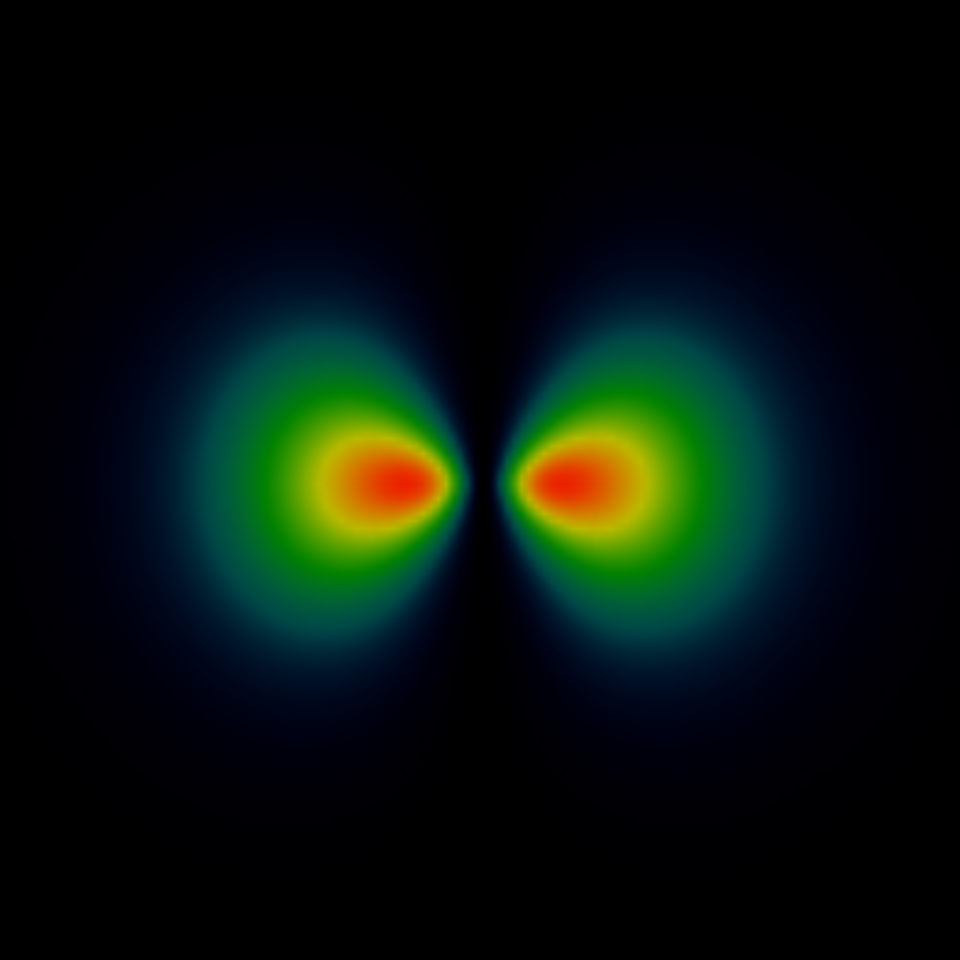
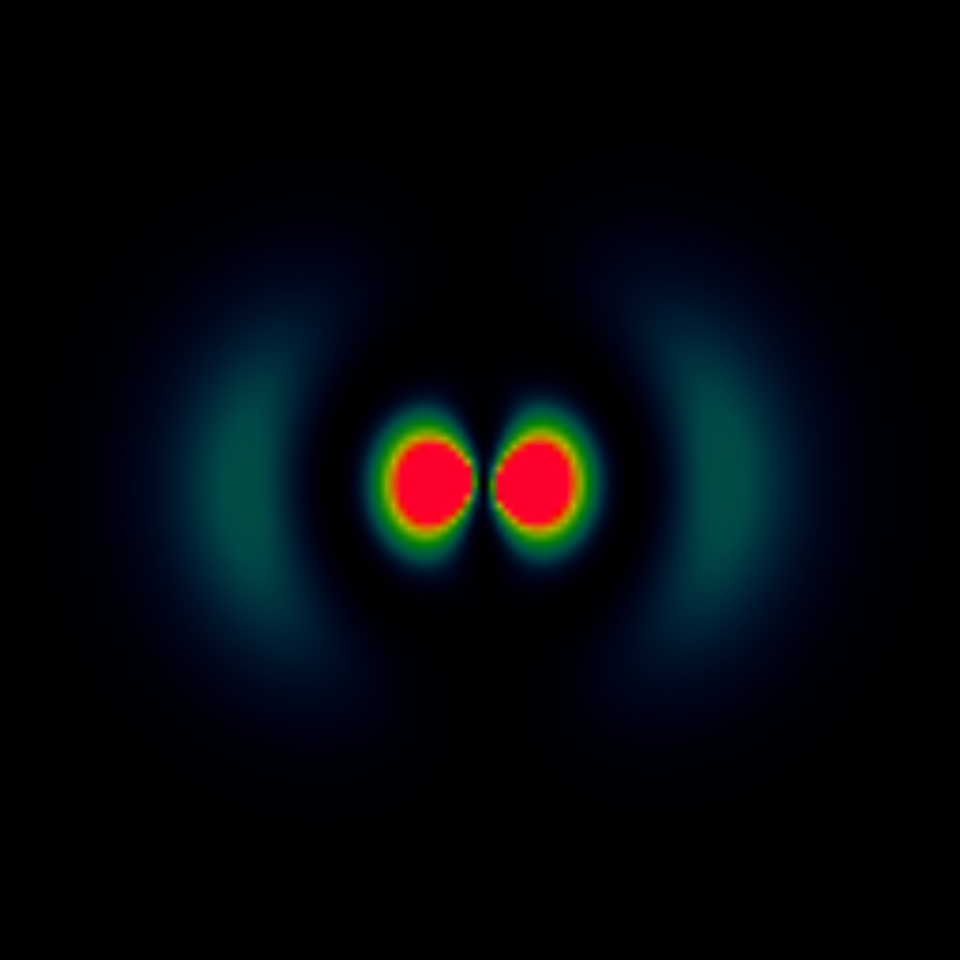
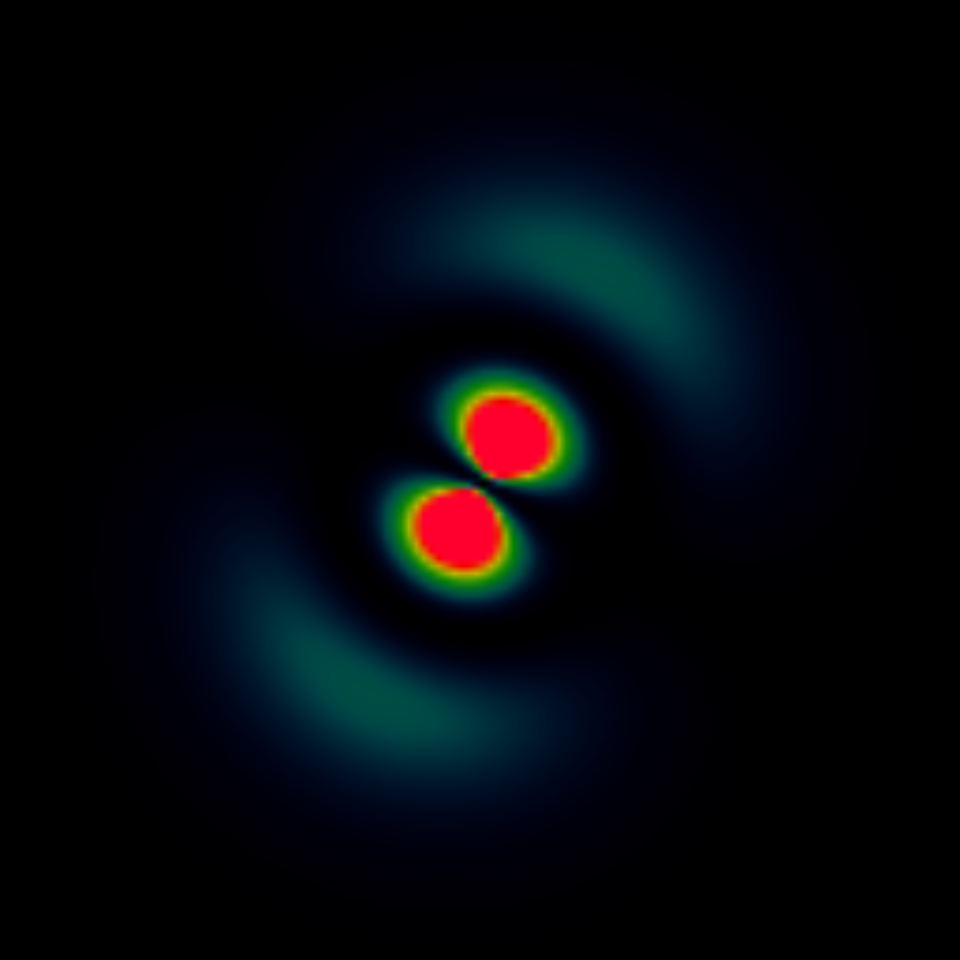
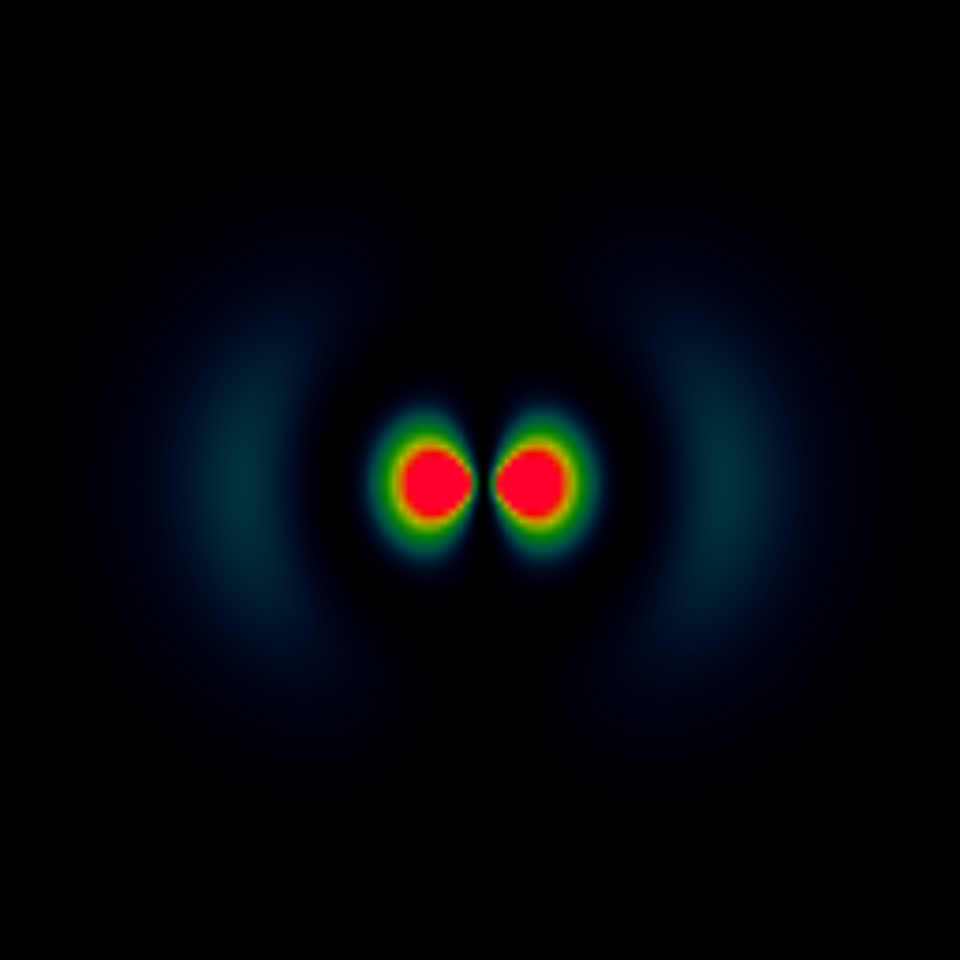
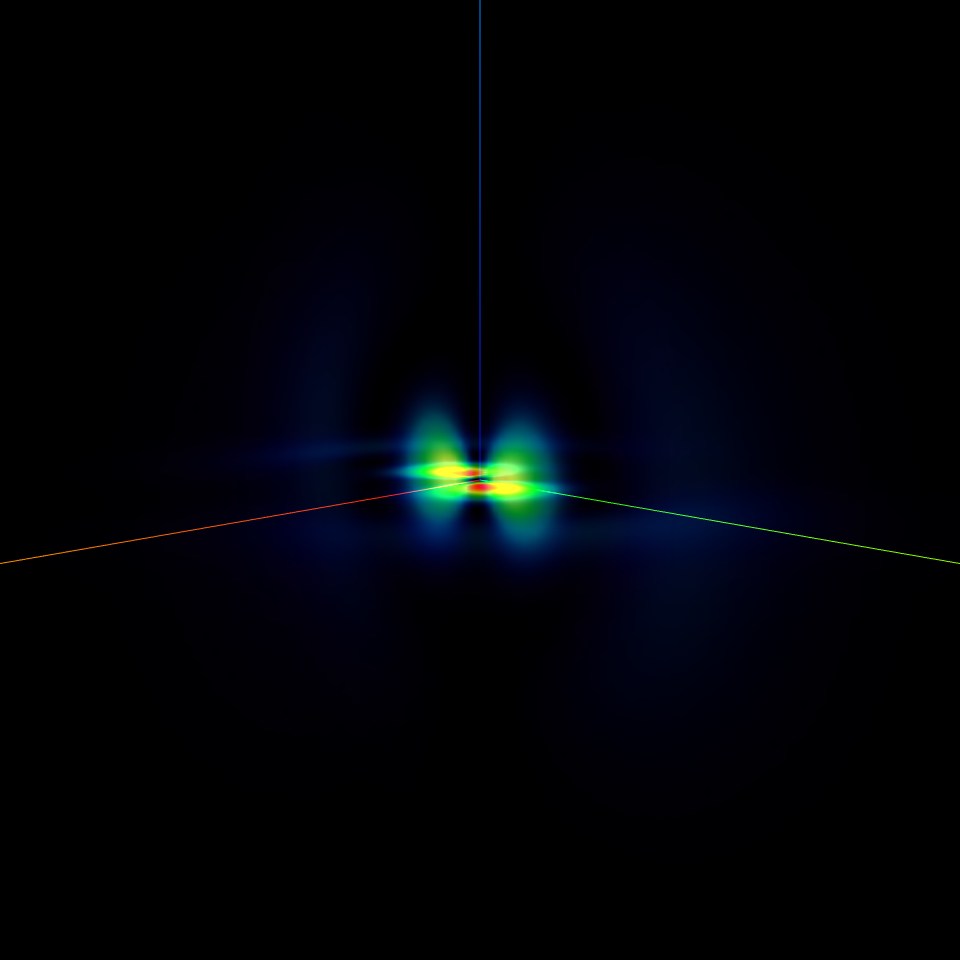
今回も独立電子近似の場合と同様、粒子の１つが $\varphi_{100}(\boldsymbol{r})|$の最も確率の高い原点（ $\boldsymbol{r}_1=0$ あるいは $\boldsymbol{r}_2=0$ ）に存在するとして、他方の粒子の空間確率密度を描画するよ。最初の表がパラヘリウム（対称関数・スピン1重項）、次の表がオルトヘリウム（反対称関数・スピン3重項）だよ。
| 記号 | $(n_1,l_1,m_1)\times(n_2,l_2,m_2)$ | 展開係数 | 対称関数 | 描画範囲 | ||||
|---|---|---|---|---|---|---|---|---|
| 実部 | 虚部 | XY平面 | YZ平面 | ZX平面 | 3面表示 | |||
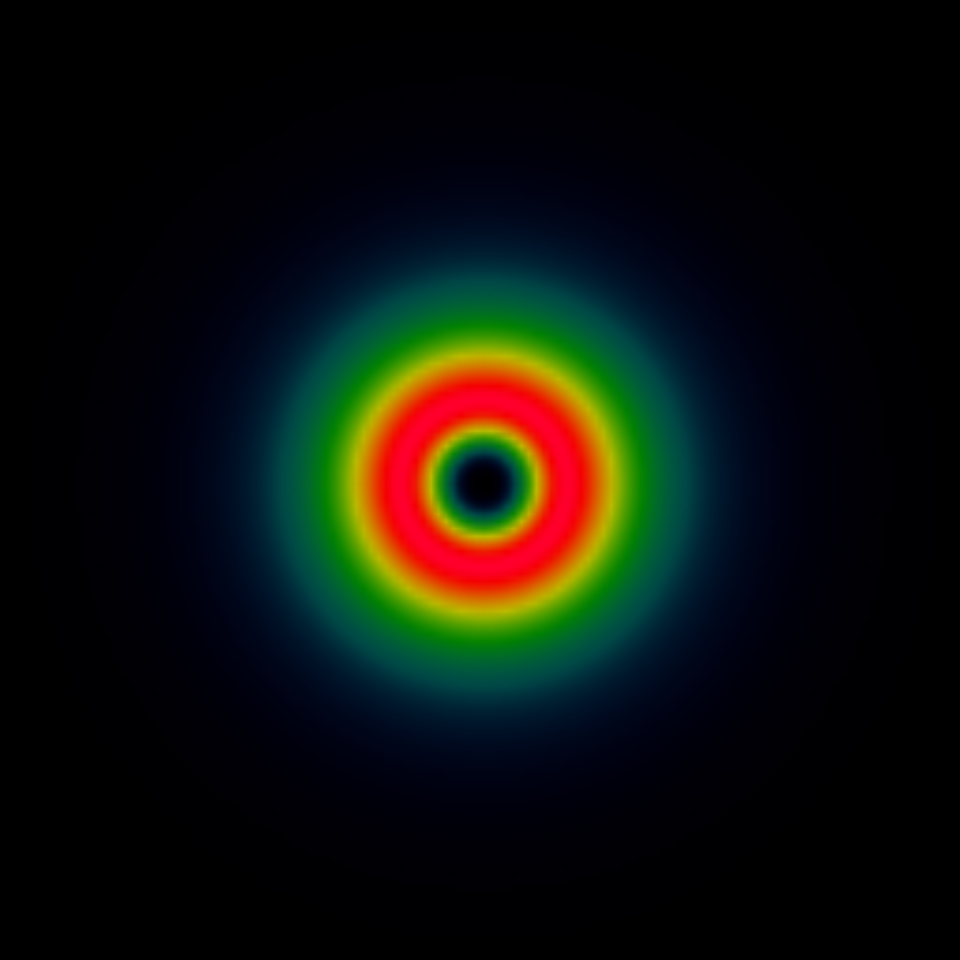
| $1s$ | $(1,0,0)\times(1,0,0)$ | 0.921 | 0 |  |
 |
 |
|
$L=2[a_B]$ |
| $(1,0,0)\times(2,0,0)$ | -0.384 | 0 | ||||||
| $2s$ | $(1,0,0)\times(1,0,0)$ | -0.114 | 0 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(2,0,0)$ | -0.606 | 0.001 | ||||||
| $(1,0,0)\times(3,0,0)$ | 0.766 | -0.004 | ||||||
| $2p$ | $(1,0,0)\times(2,1,-1)$ | 0.194 | -0.339 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(2,1,0)$ | -0.155 | -0.528 | ||||||
| $(1,0,0)\times(2,1,1)$ | 0.064 | 0.0825 | ||||||
| $(1,0,0)\times(3,1,-1)$ | -0.211 | 0.358 | ||||||
| $(1,0,0)\times(3,1,0)$ | 0.162 | -0.561 | ||||||
| $(1,0,0)\times(3,1,1)$ | -0.0629 | -0.081 | ||||||
| $2p$ | $(1,0,0)\times(2,1,-1)$ | -0.463 | -0.058 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(2,1,0)$ | -0.278 | 0.087 | ||||||
| $(1,0,0)\times(2,1,1)$ | -0.379 | 0.101 | ||||||
| $(1,0,0)\times(3,1,-1)$ | 0.508 | 0.065 | ||||||
| $(1,0,0)\times(3,1,0)$ | 0.304 | -0.094 | ||||||
| $(1,0,0)\times(3,1,1)$ | 0.401 | -0.116 | ||||||
| $2p$ | $(1,0,0)\times(2,1,-1)$ | 0.267 | 0.125 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(2,1,0)$ | 0.241 | 0.134 | ||||||
| $(1,0,0)\times(2,1,1)$ | -0.527 | -0.119 | ||||||
| $(1,0,0)\times(3,1,-1)$ | 0.290 | -0.127 | ||||||
| $(1,0,0)\times(3,1,0)$ | -0.259 | -0.151 | ||||||
| $(1,0,0)\times(3,1,1)$ | 0.565 | 0.136 | ||||||
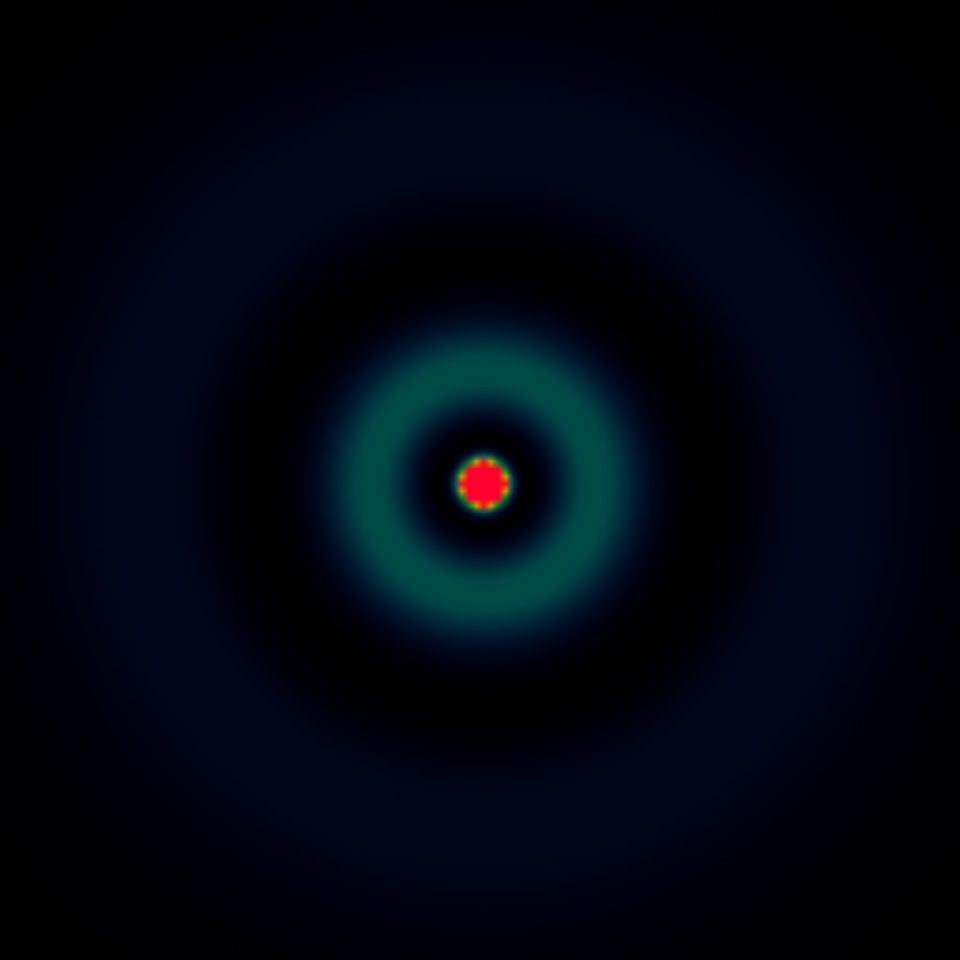
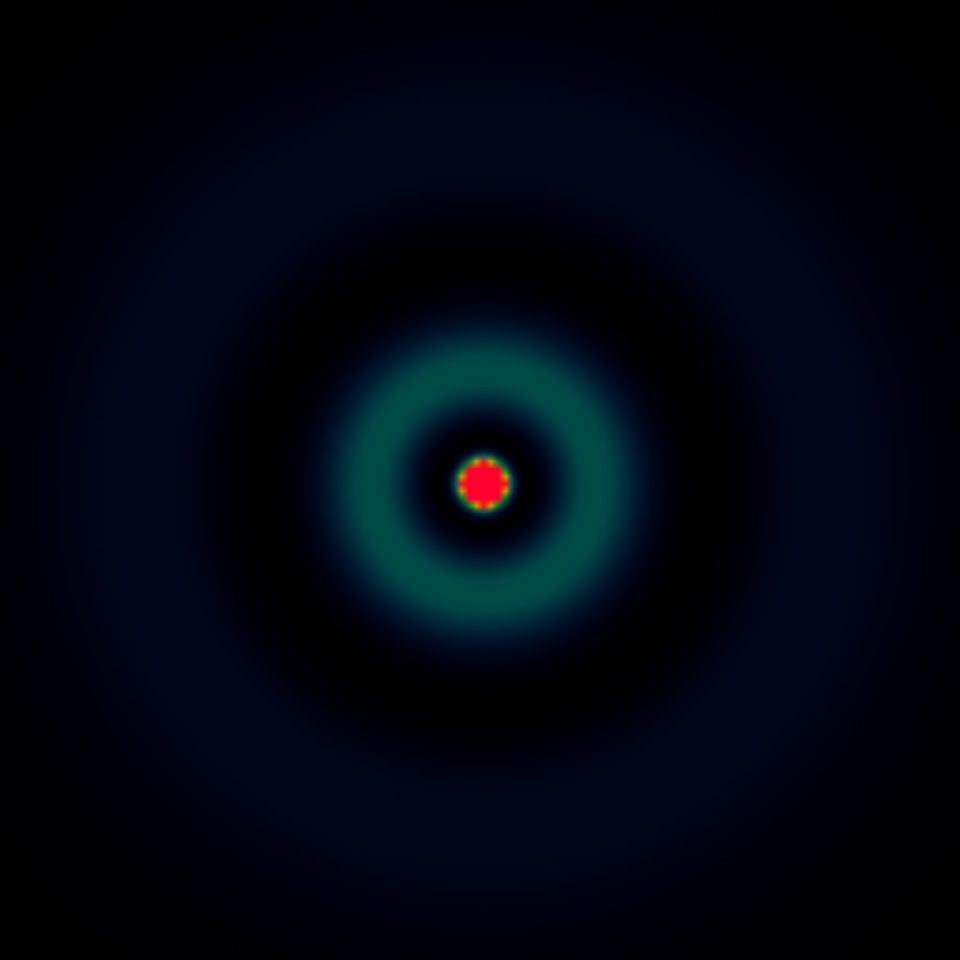
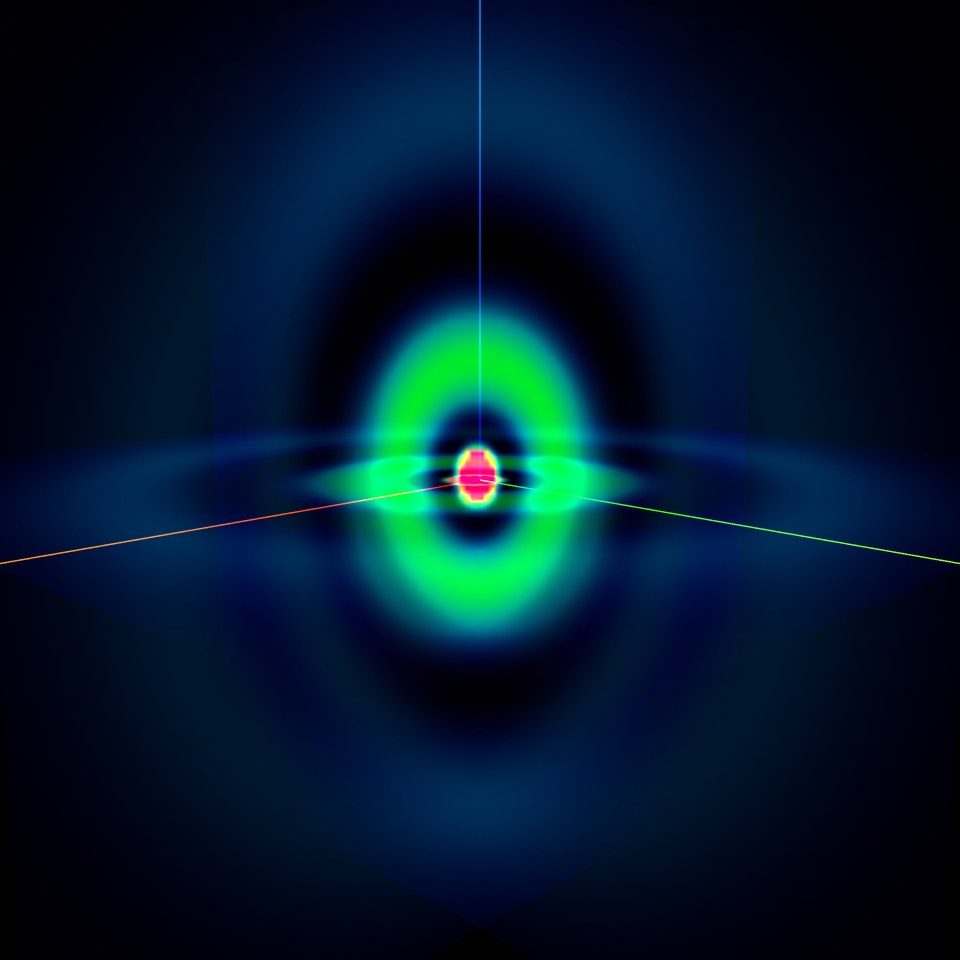
| $3s$ | - | - | - |  |
 |
 |
|
$L=30[a_B]$ |
| $3p$ | - | - | - |  |
 |
 |
|
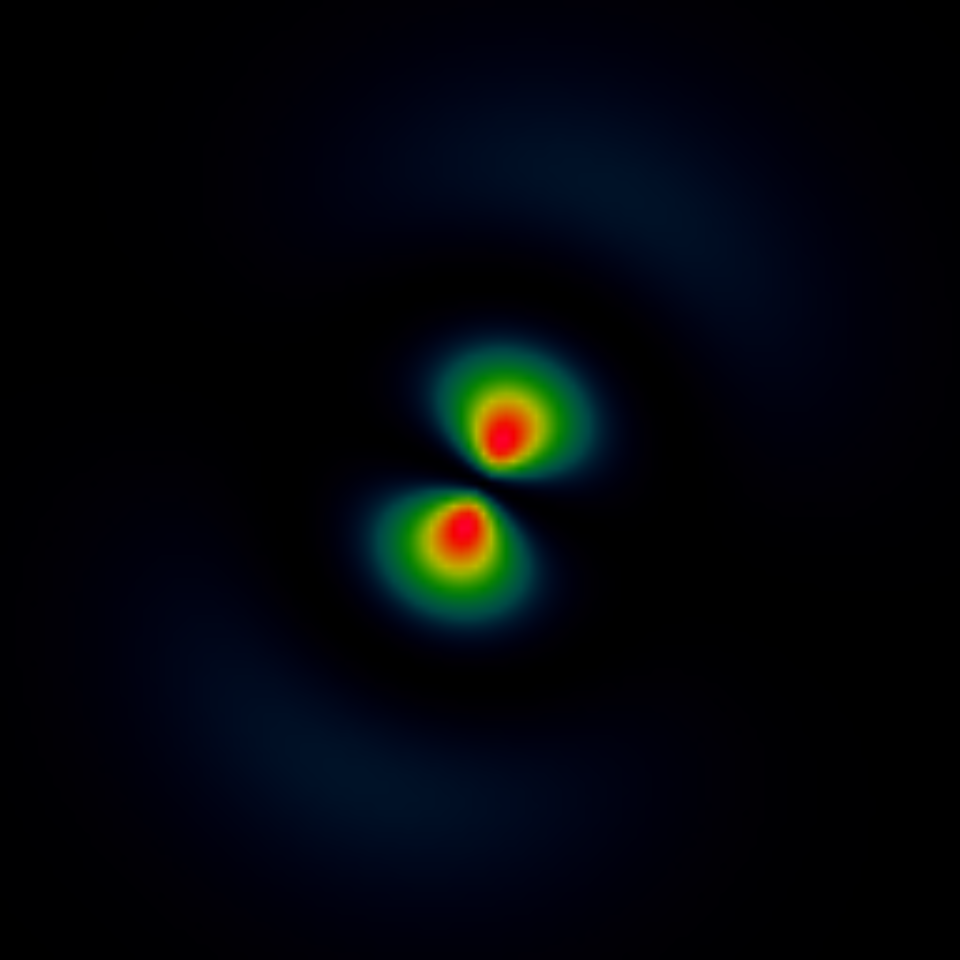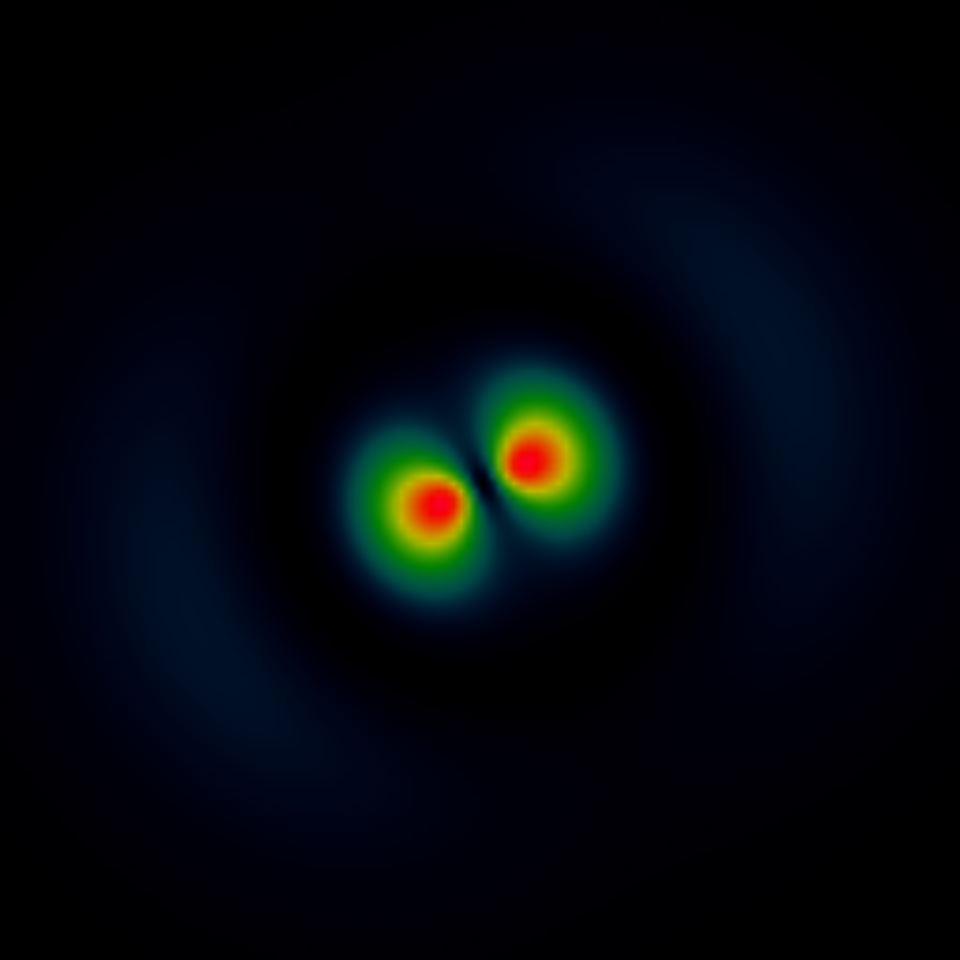$L=30[a_B]$ |
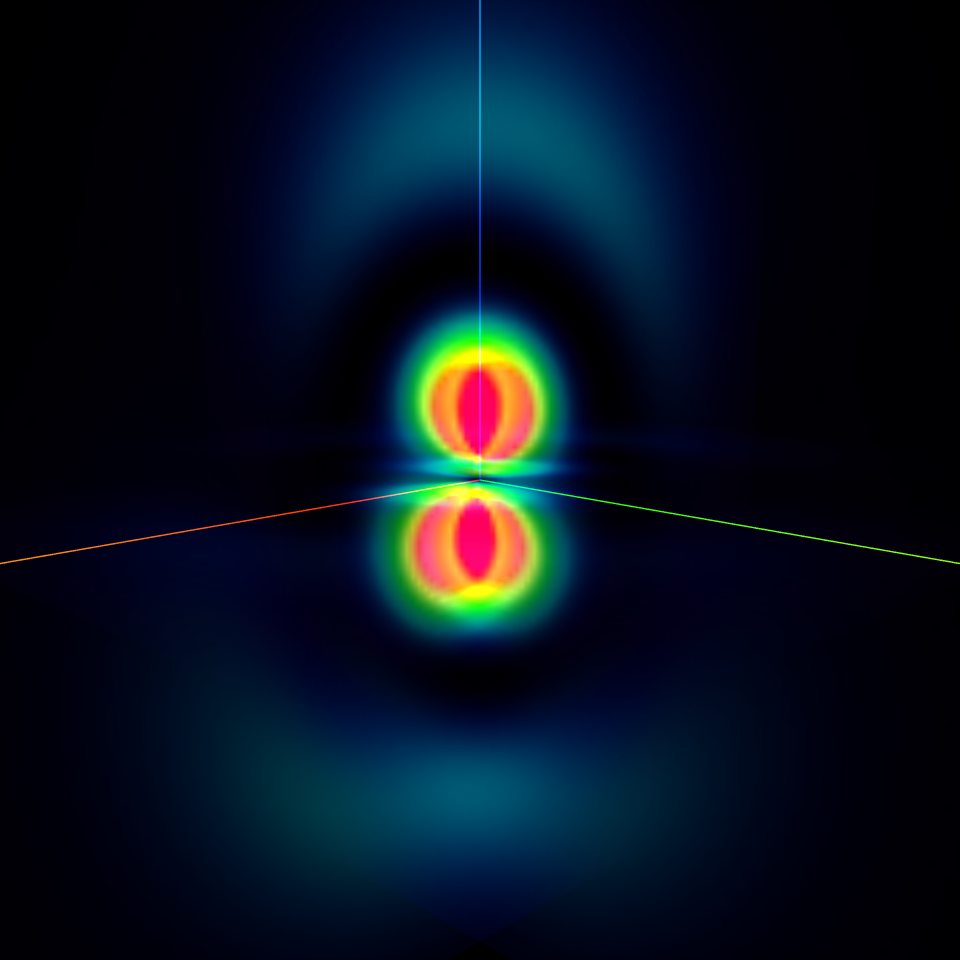
| $3p$ | - | - | - |  |
 |
 |
|
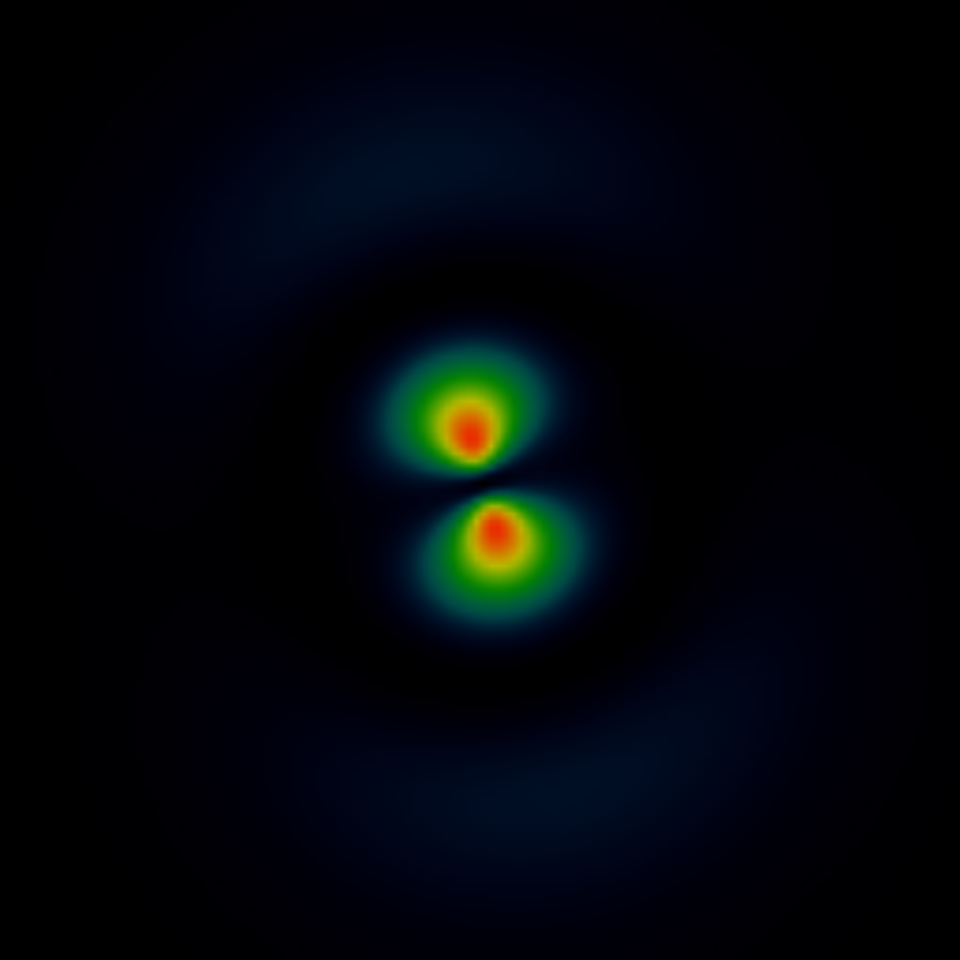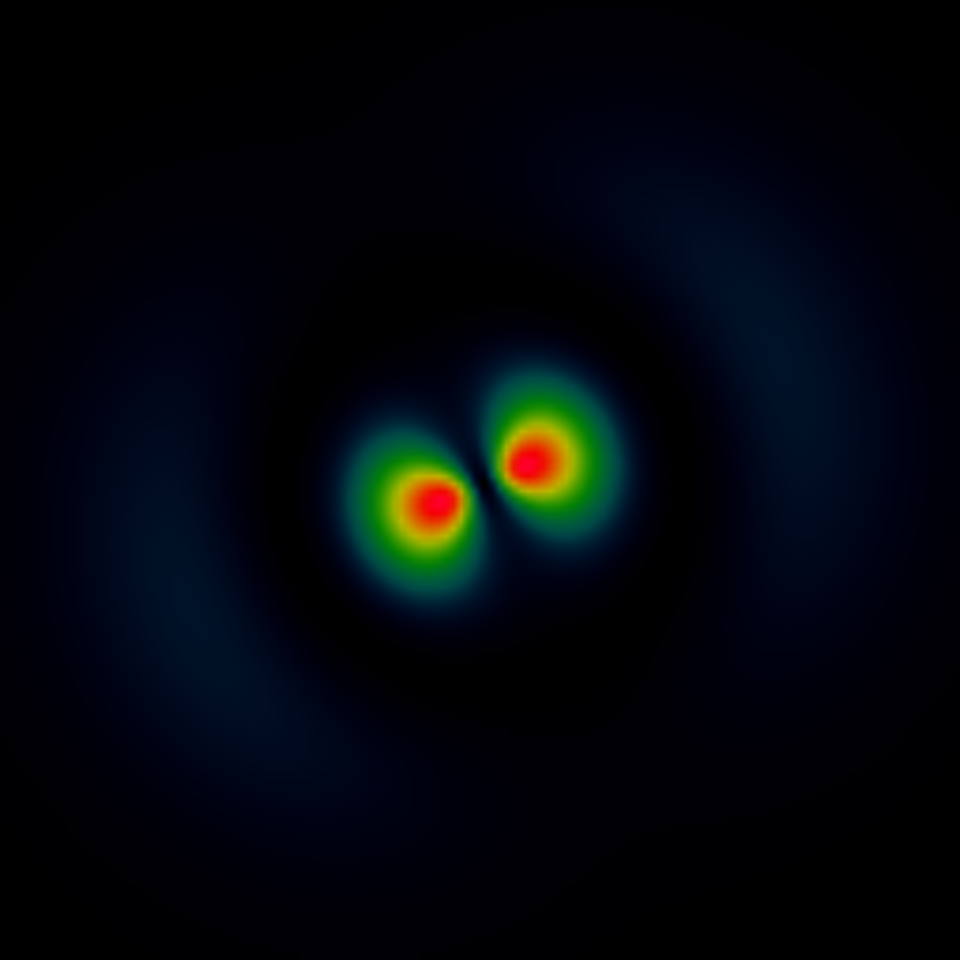$L=30[a_B]$ |
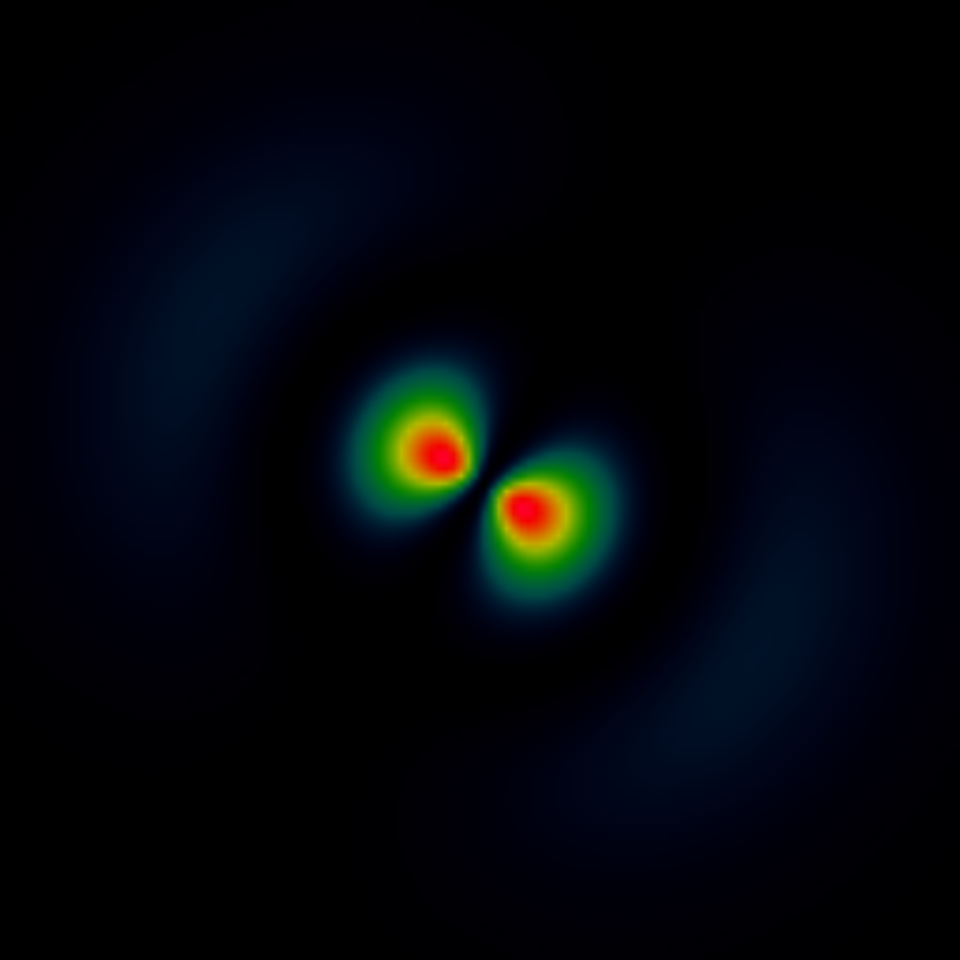
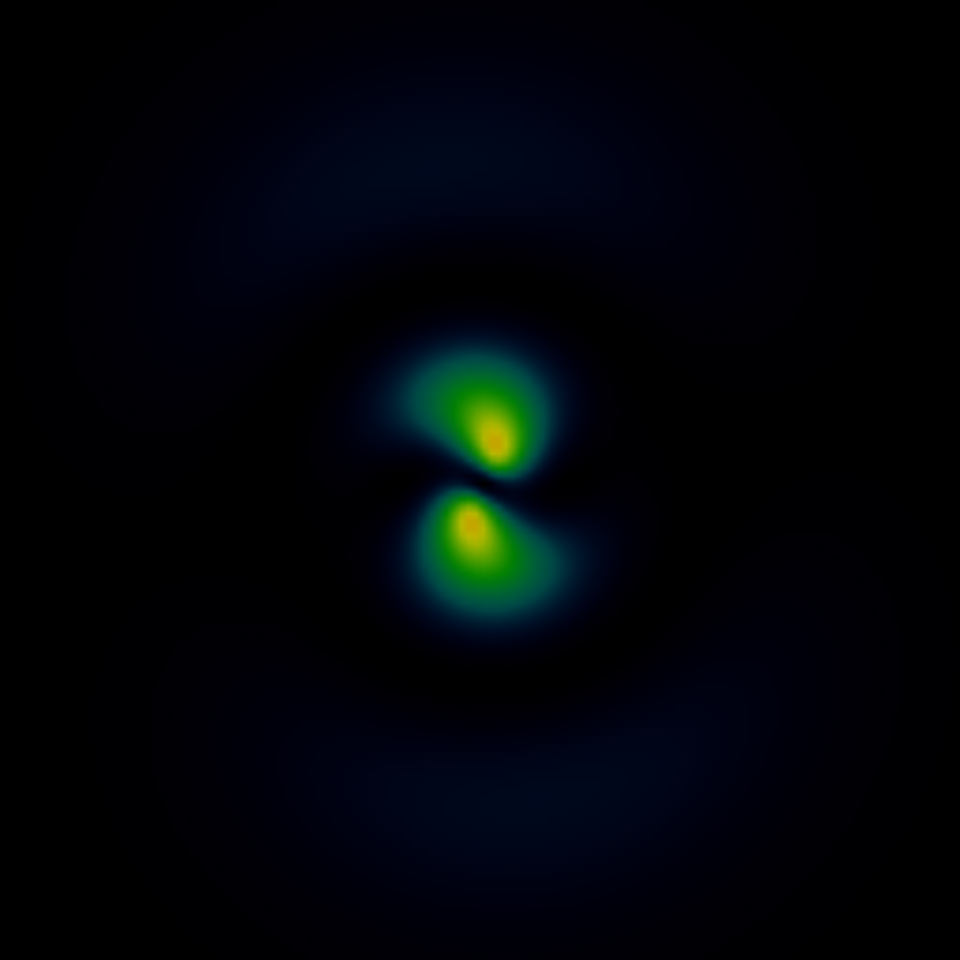
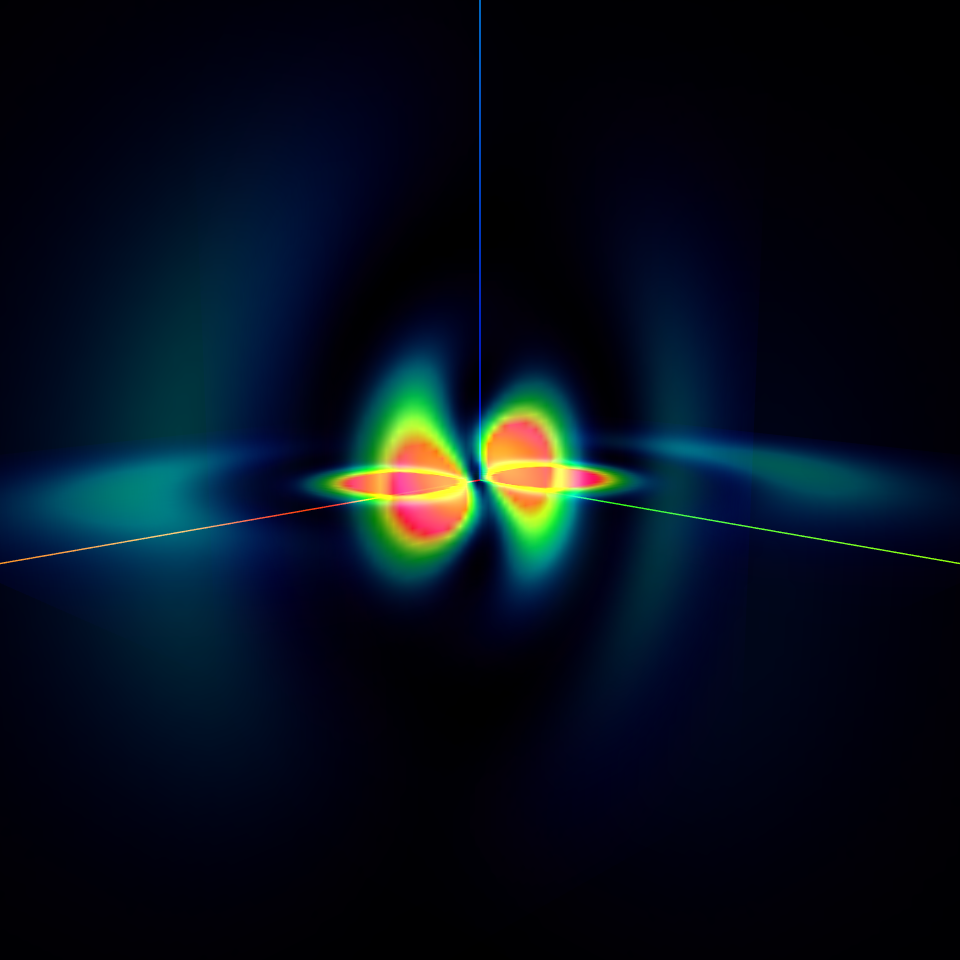
| $3p$ | - | - | - |  |
 |
 |
|
$L=30[a_B]$ |
| $3d$ | - | - | - |  |
 |
 |
|
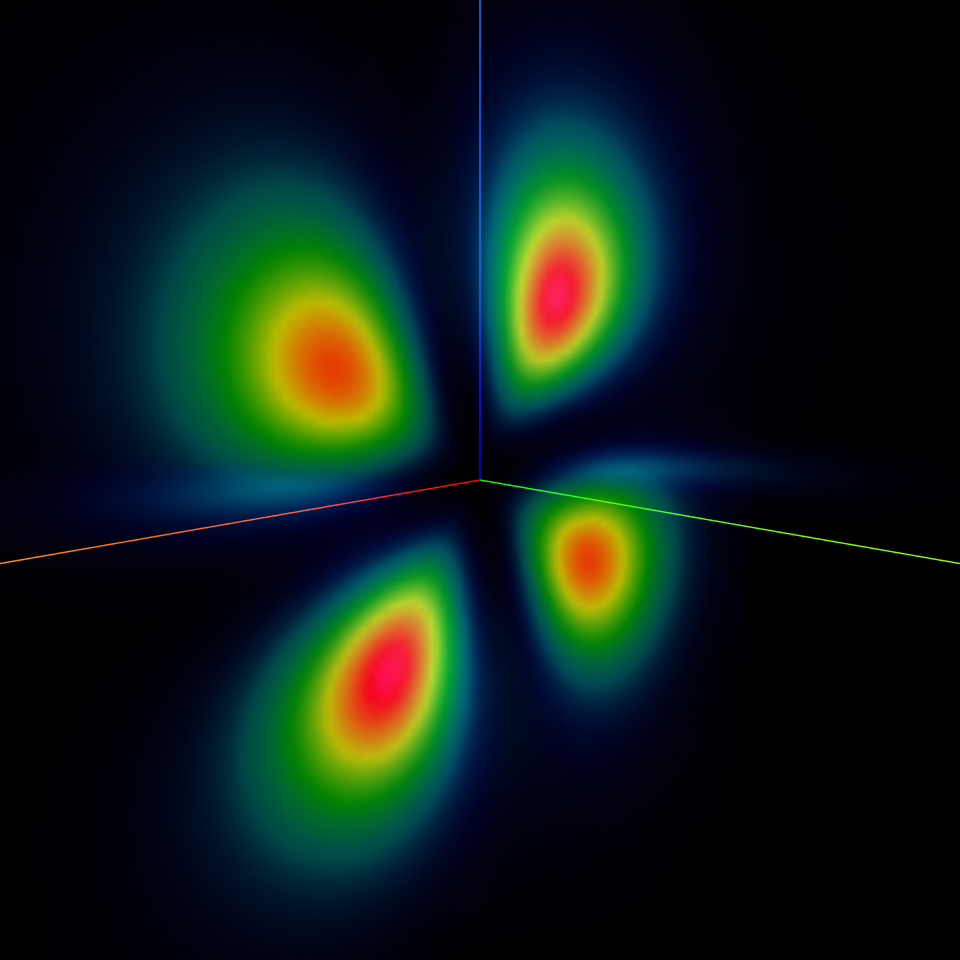
$L=30[a_B]$ |
| $3d$ | - | - | - |  |
 |
 |
|
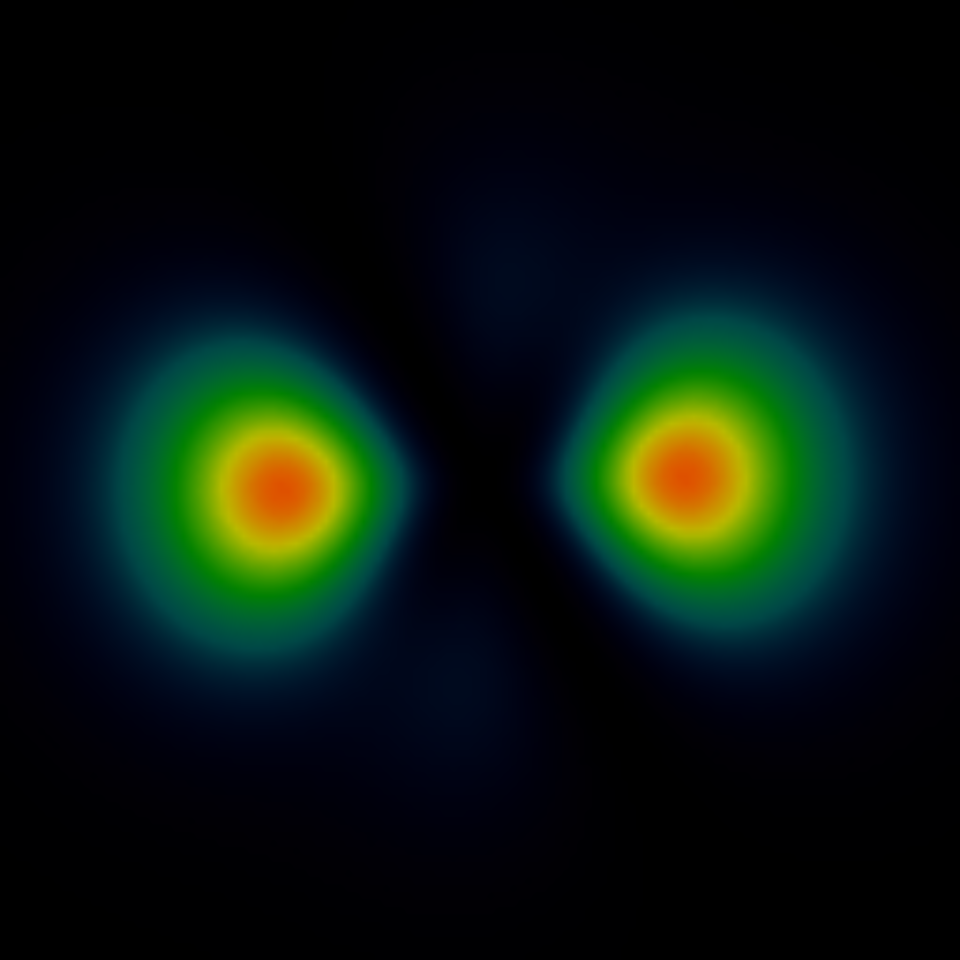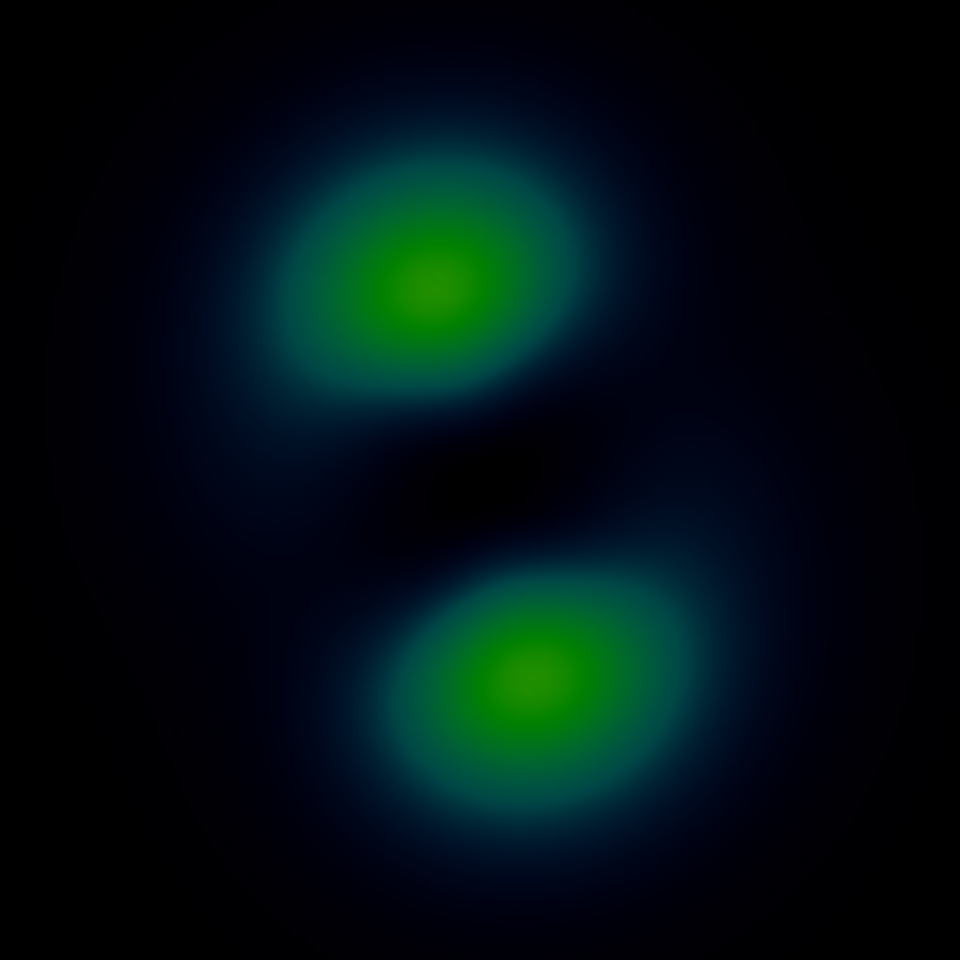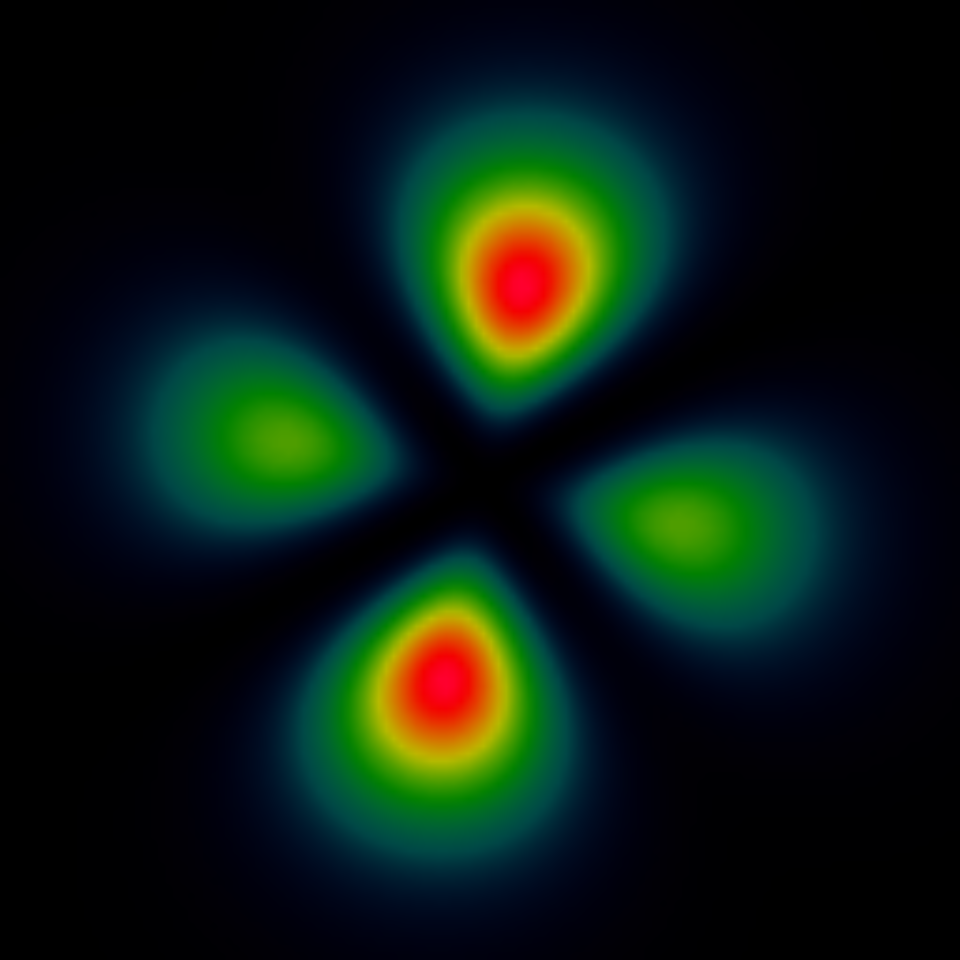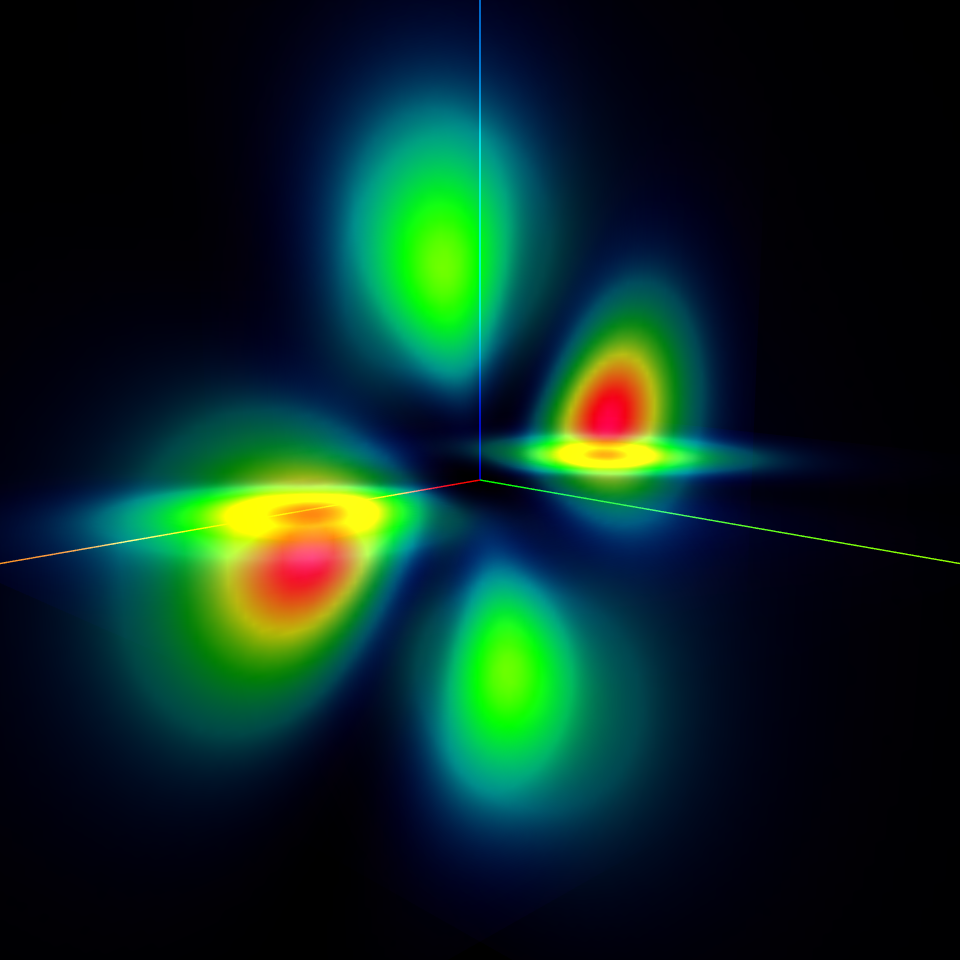
$L=30[a_B]$ |
| $3d$ | - | - | - |  |
 |
 |
|
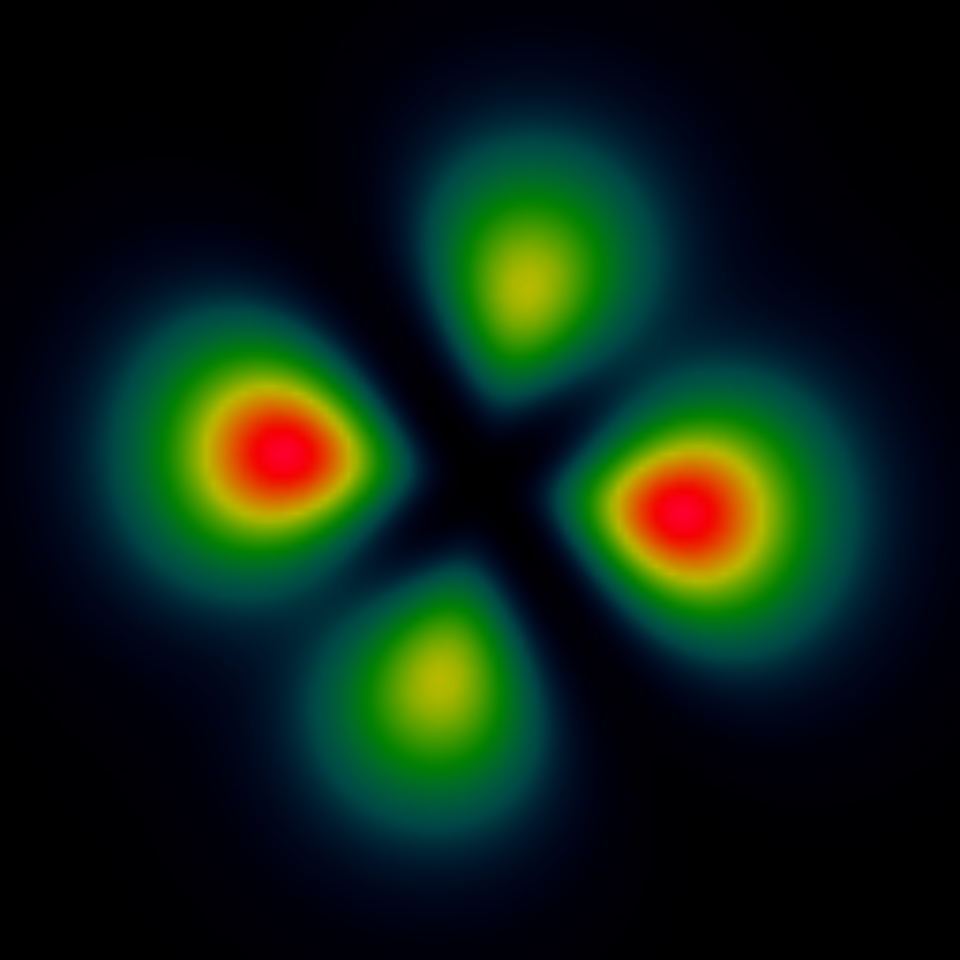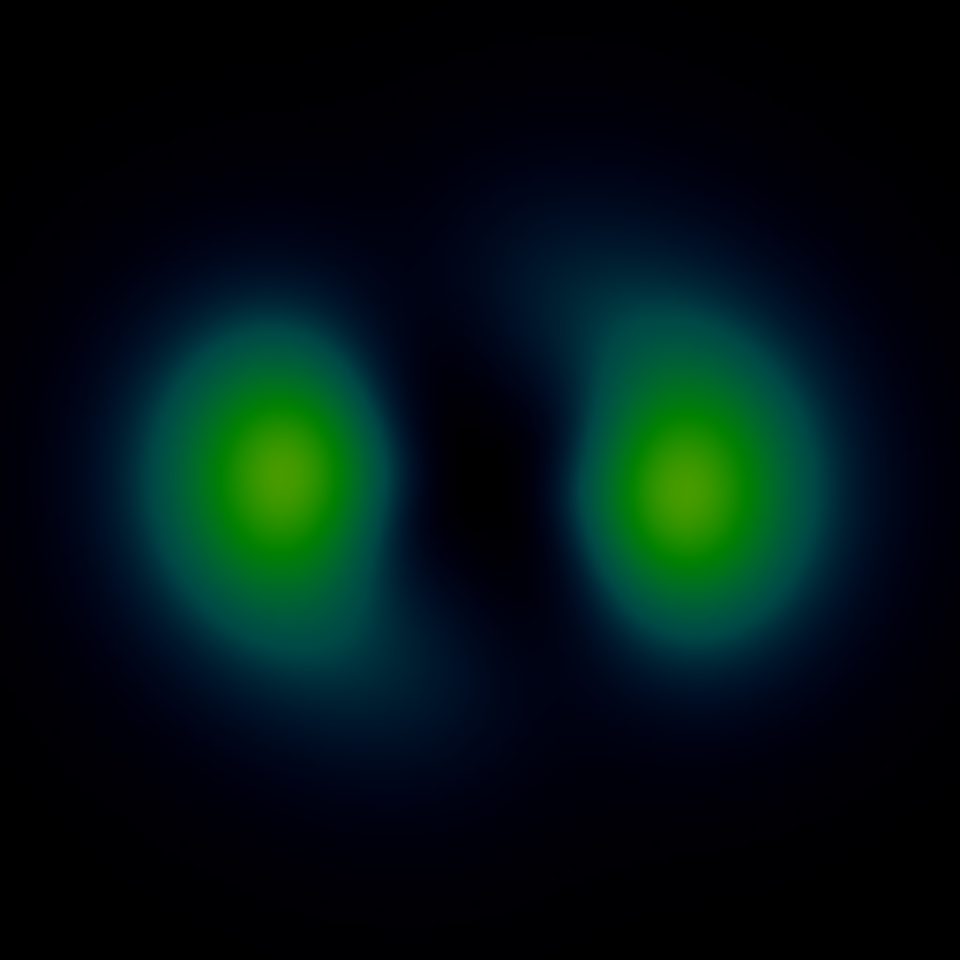$L=30[a_B]$ |
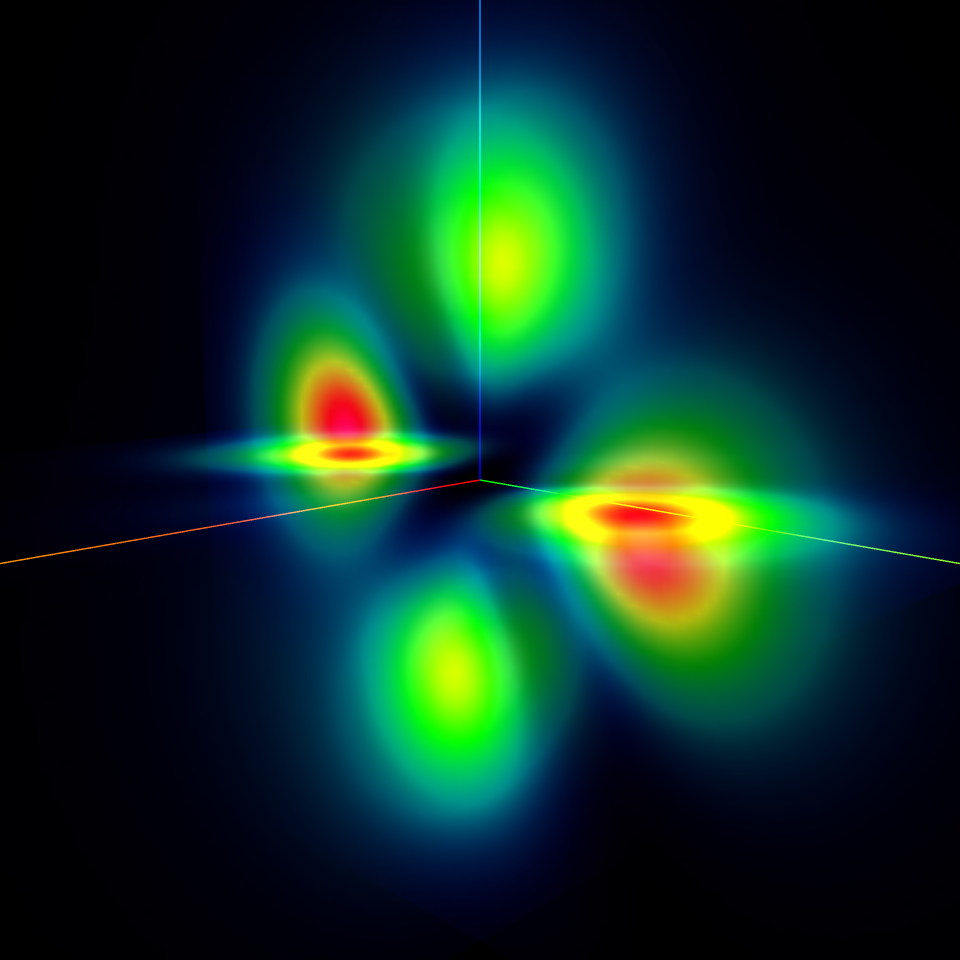
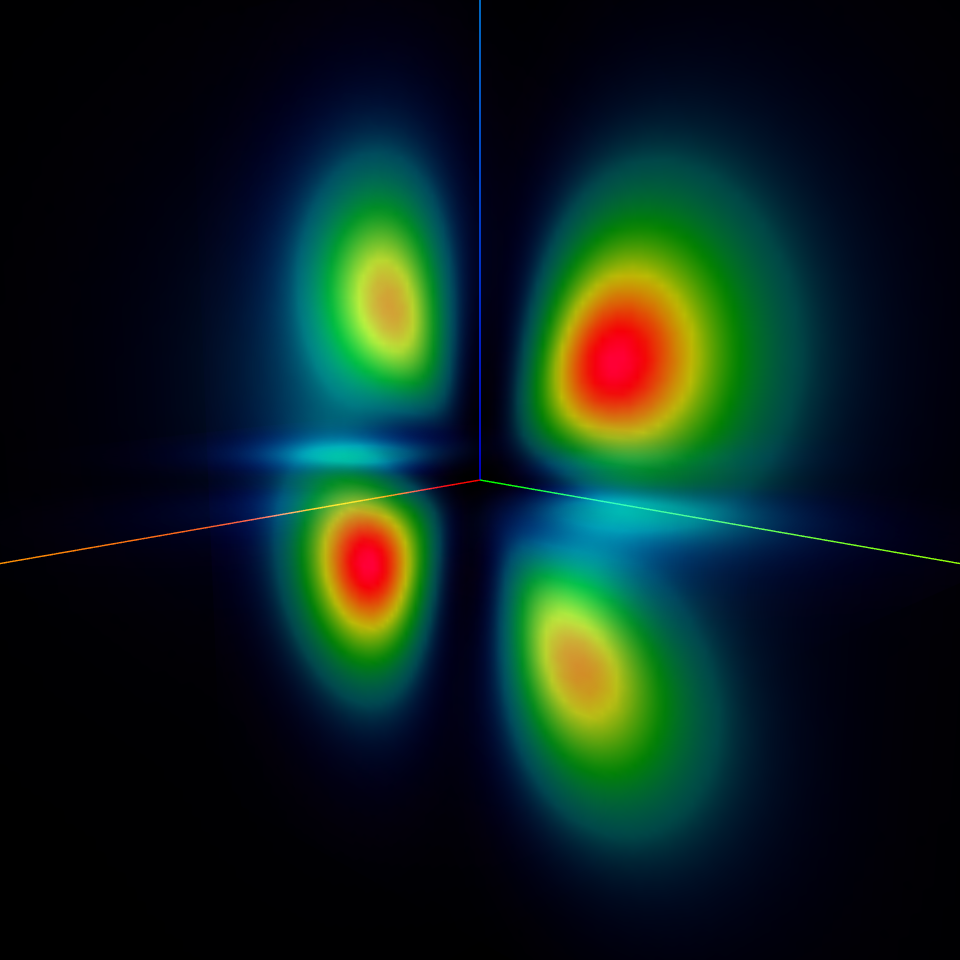
| $3d$ | - | - | - |  |
 |
 |
|
$L=30[a_B]$ |
| $3d$ | - | - | - |  |
 |
 |
|
$L=30[a_B]$ |
| 記号 | $(n_1,l_1,m_1)\times(n_2,l_2,m_2)$ | 展開係数 | 反対称関数 | 描画範囲 | ||||
|---|---|---|---|---|---|---|---|---|
| 実部 | 虚部 | XY平面 | YZ平面 | ZX平面 | 3面表示 | |||
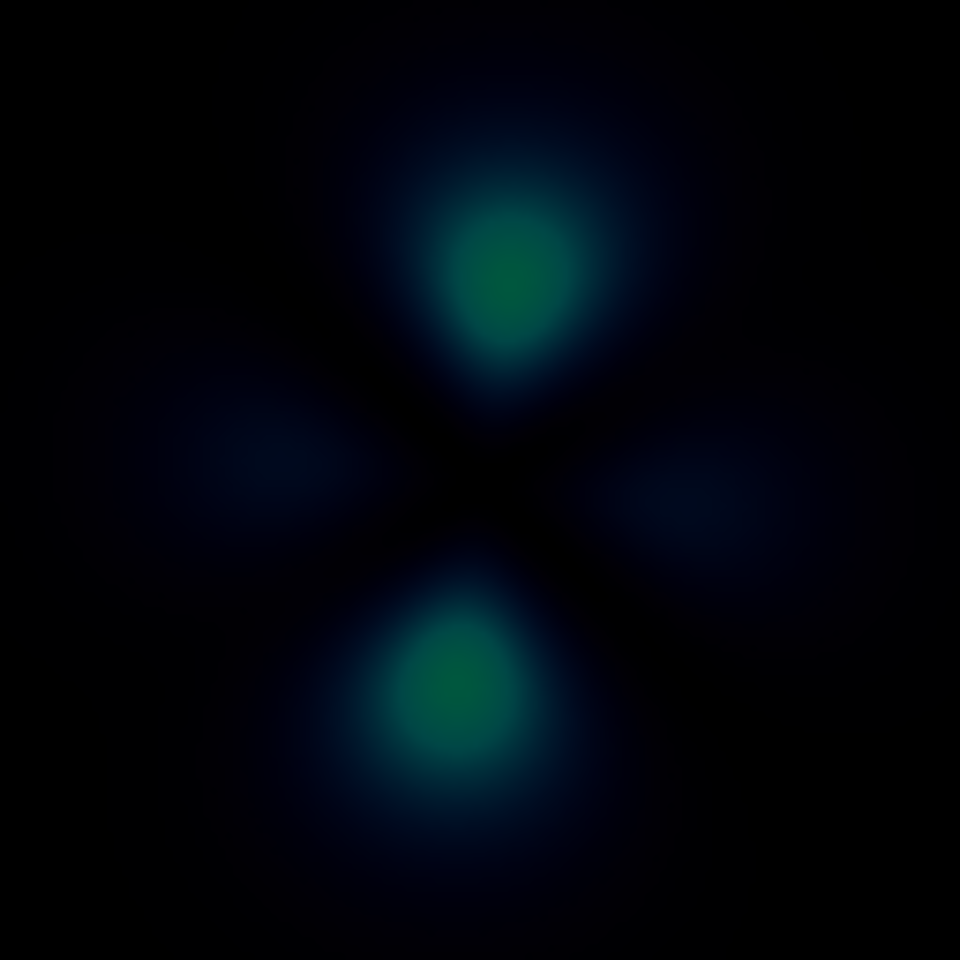
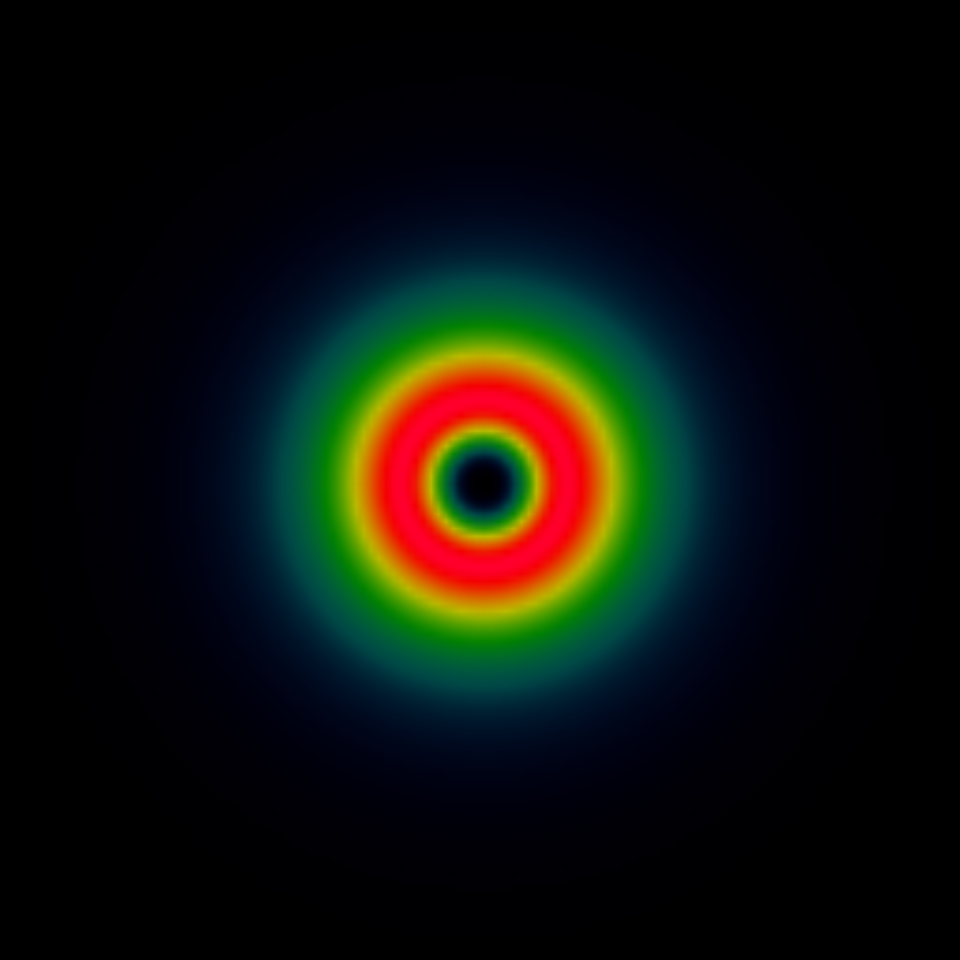
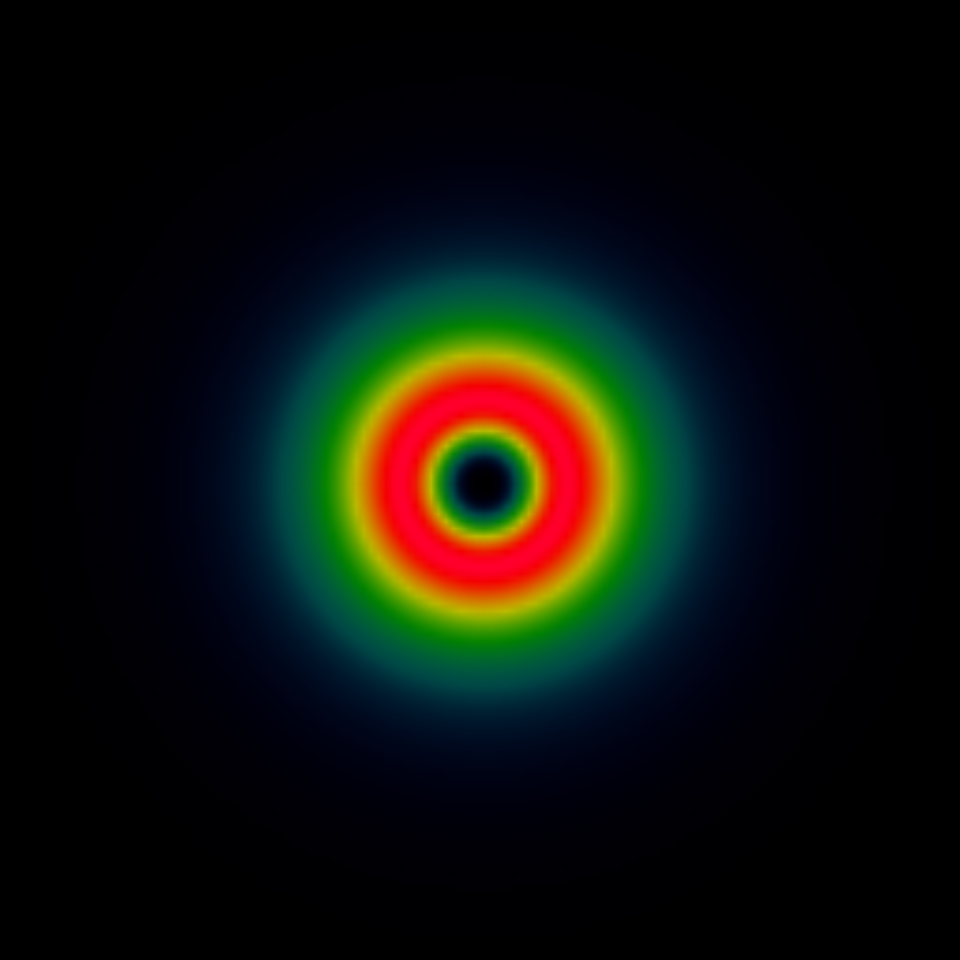
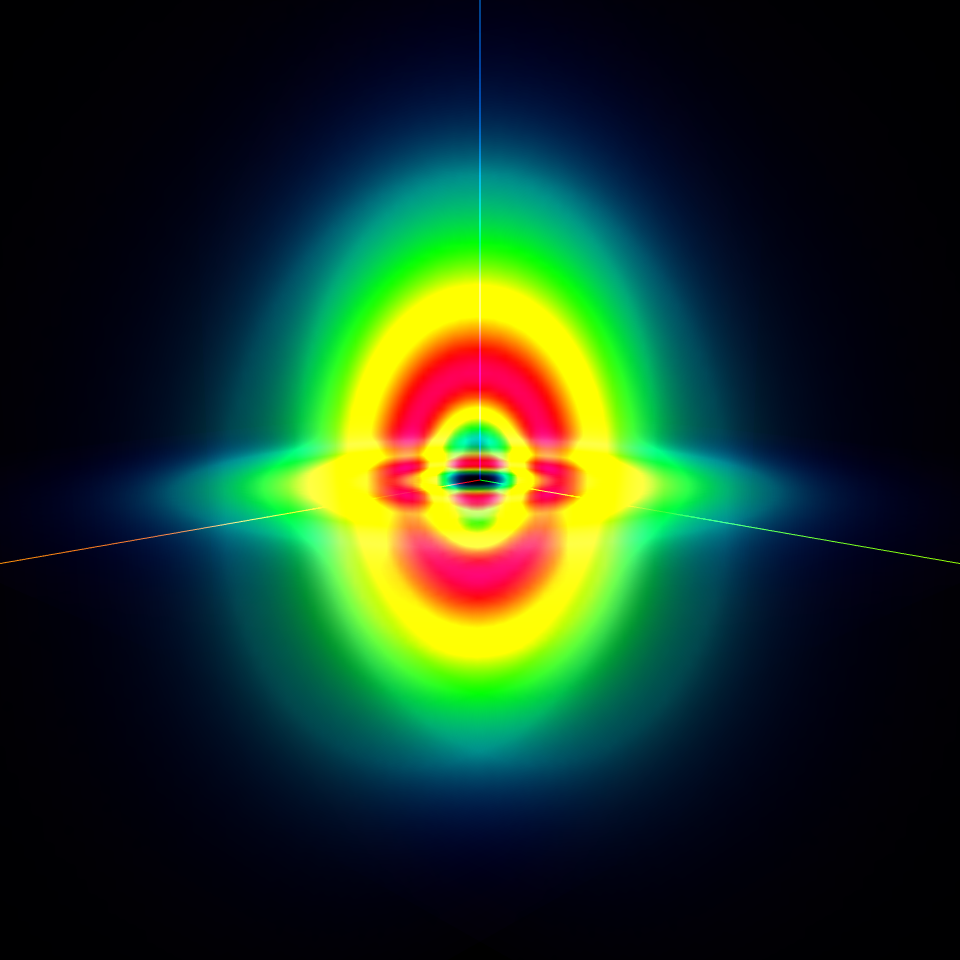
| $2s$ | $(1,0,0)\times(2,0,0)$ | 0.875 | 0 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(3,0,0)$ | -0.469 | 0 | ||||||
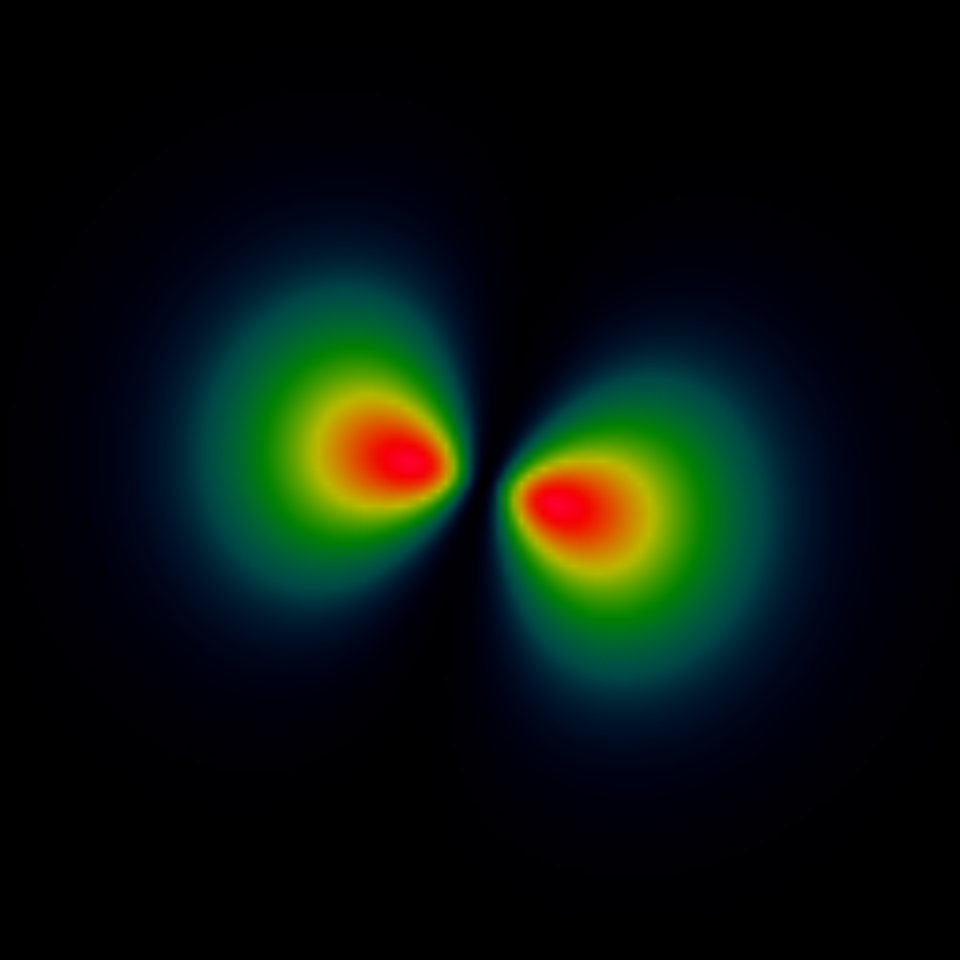
| $2p$ | $(1,0,0)\times(2,1,-1)$ | -0.101 | 0.300 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(2,1,0)$ | 0.404 | -0.309 | ||||||
| $(1,0,0)\times(2,1,1)$ | 0.005 | 0.492 | ||||||
| $(1,0,0)\times(3,1,-1)$ | 0.0790 | -0.245 | ||||||
| $(1,0,0)\times(3,1,0)$ | -0.335 | 0.251 | ||||||
| $(1,0,0)\times(3,1,1)$ | 0.002 | -0.393 | ||||||
| $2p$ | $(1,0,0)\times(2,1,-1)$ | 0.336 | 0.596 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(2,1,1)$ | -0.254 | -0.255 | ||||||
| $(1,0,0)\times(3,1,-1)$ | -0.268 | -0.478 | ||||||
| $(1,0,0)\times(3,1,1)$ | 0.2145 | 0.206 | ||||||
| $2p$ | $(1,0,0)\times(2,1,-1)$ | 0.141 | 0.130 |  |
 |
 |
|
$L=20[a_B]$ |
| $(1,0,0)\times(2,1,0)$ | 0.087 | 0.570 | ||||||
| $(1,0,0)\times(2,1,1)$ | 0.416 | 0.234 | ||||||
| $(1,0,0)\times(3,1,-1)$ | -0.118 | -0.108 | ||||||
| $(1,0,0)\times(3,1,0)$ | -0.0744 | -0.466 | ||||||
| $(1,0,0)\times(3,1,1)$ | -0.336 | -0.197 | ||||||
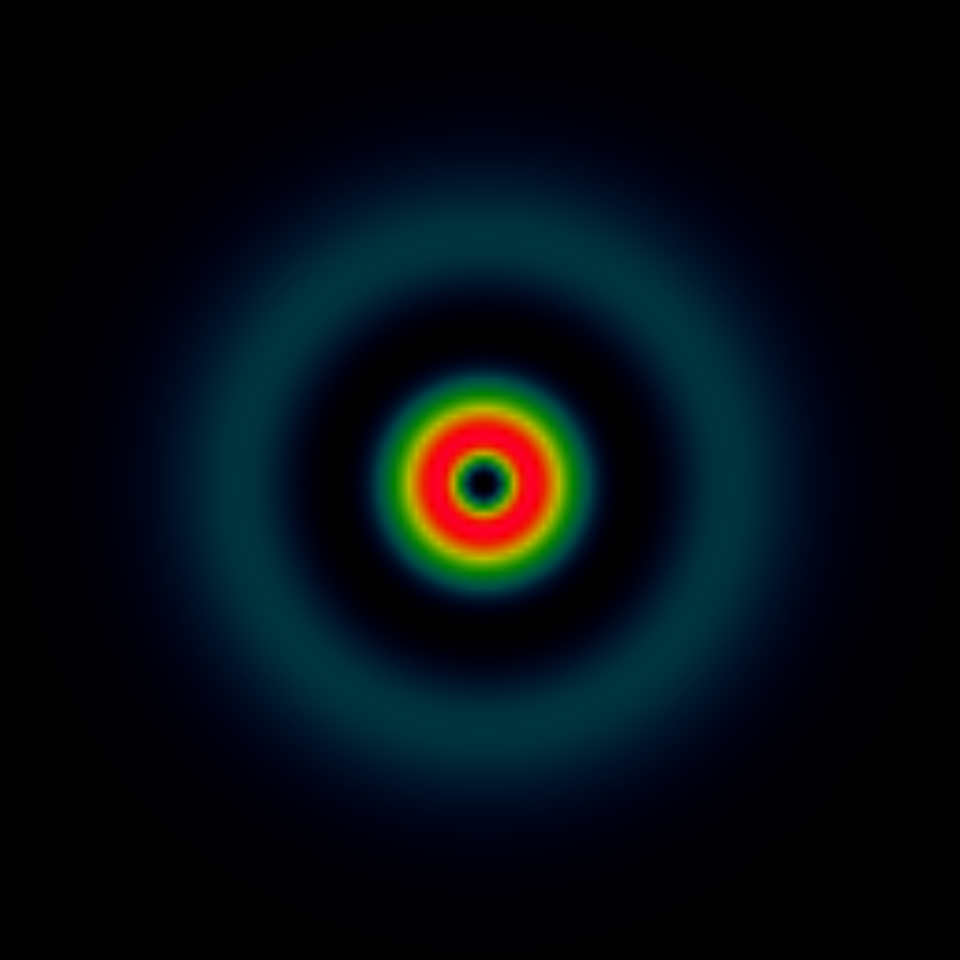
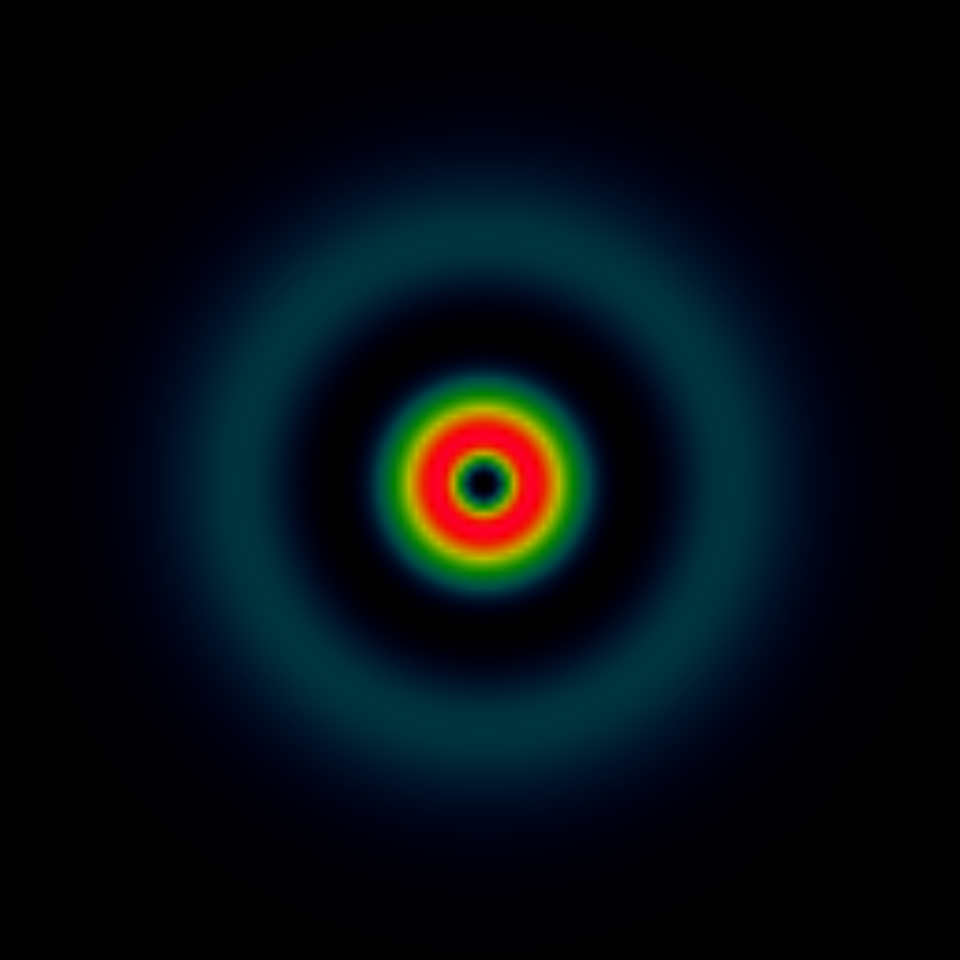
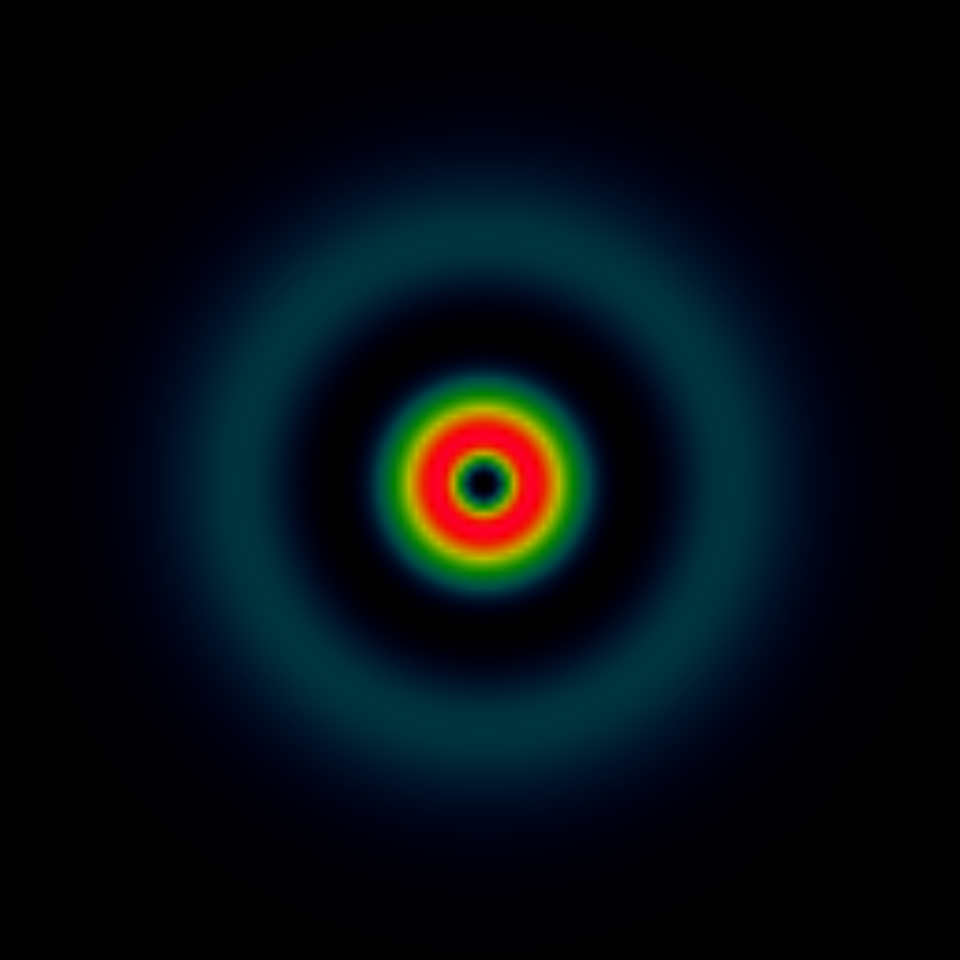
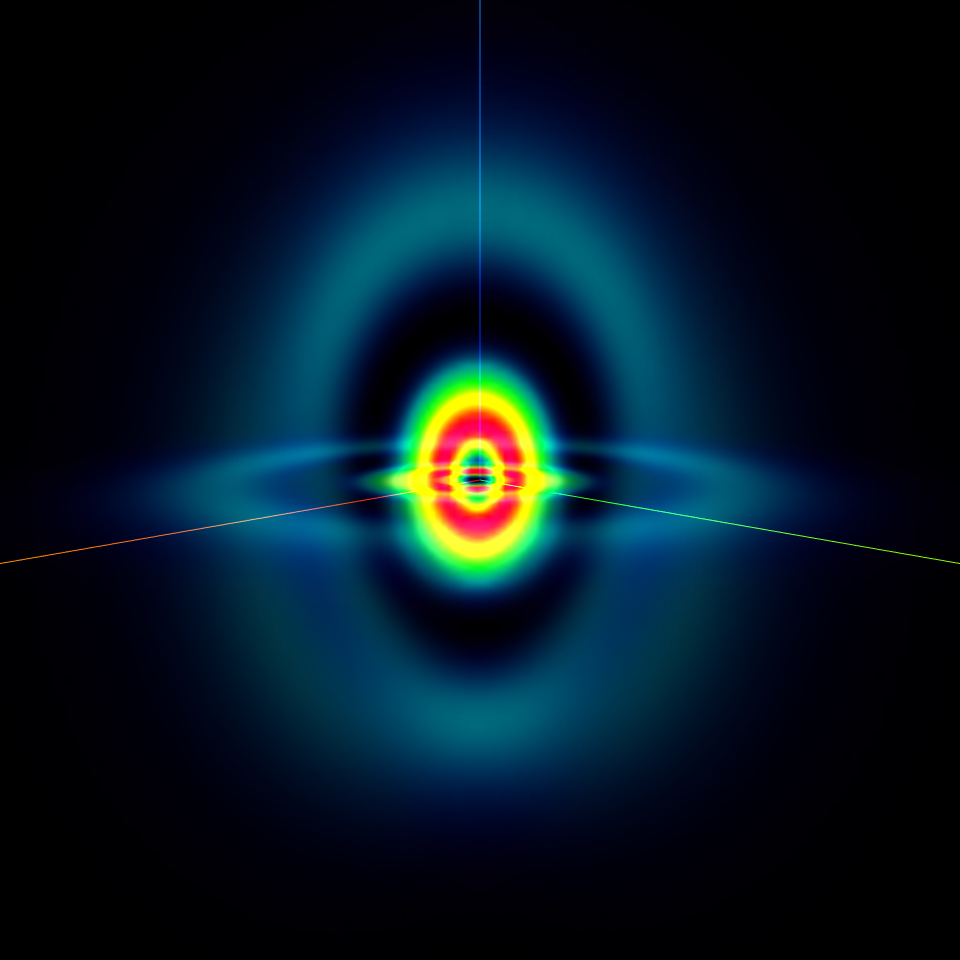
| $3s$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
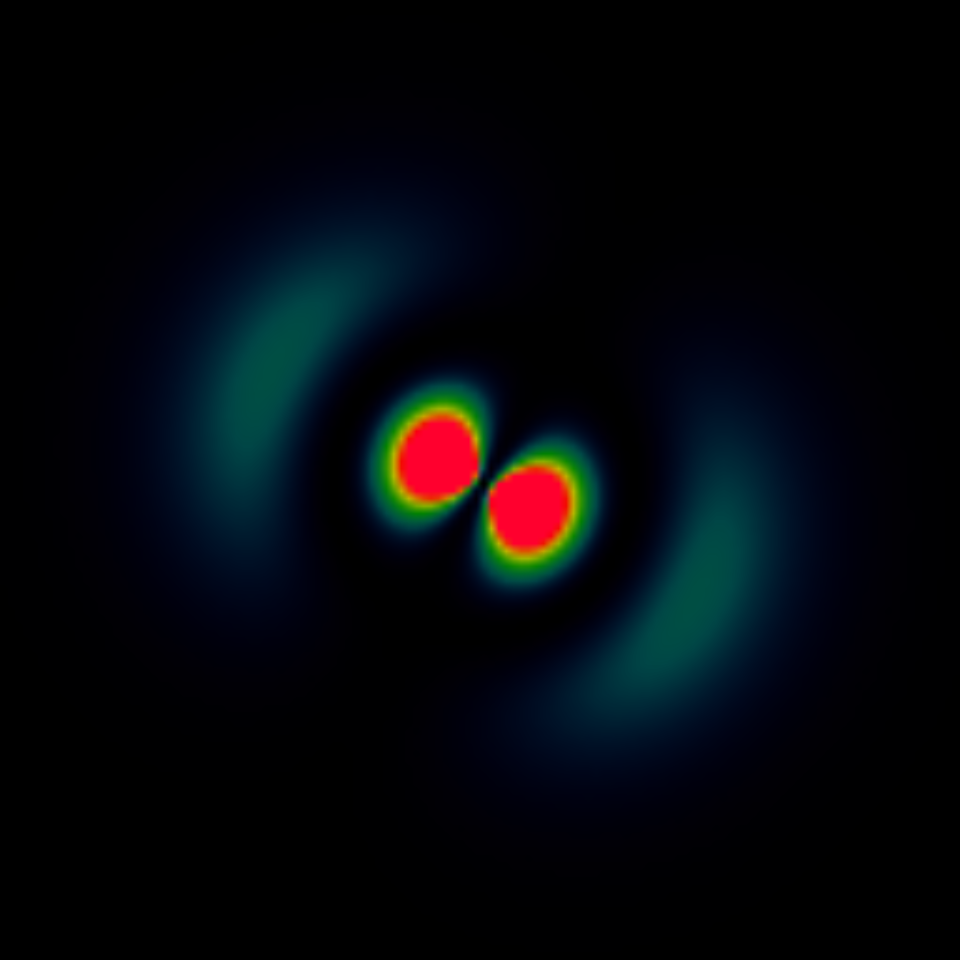
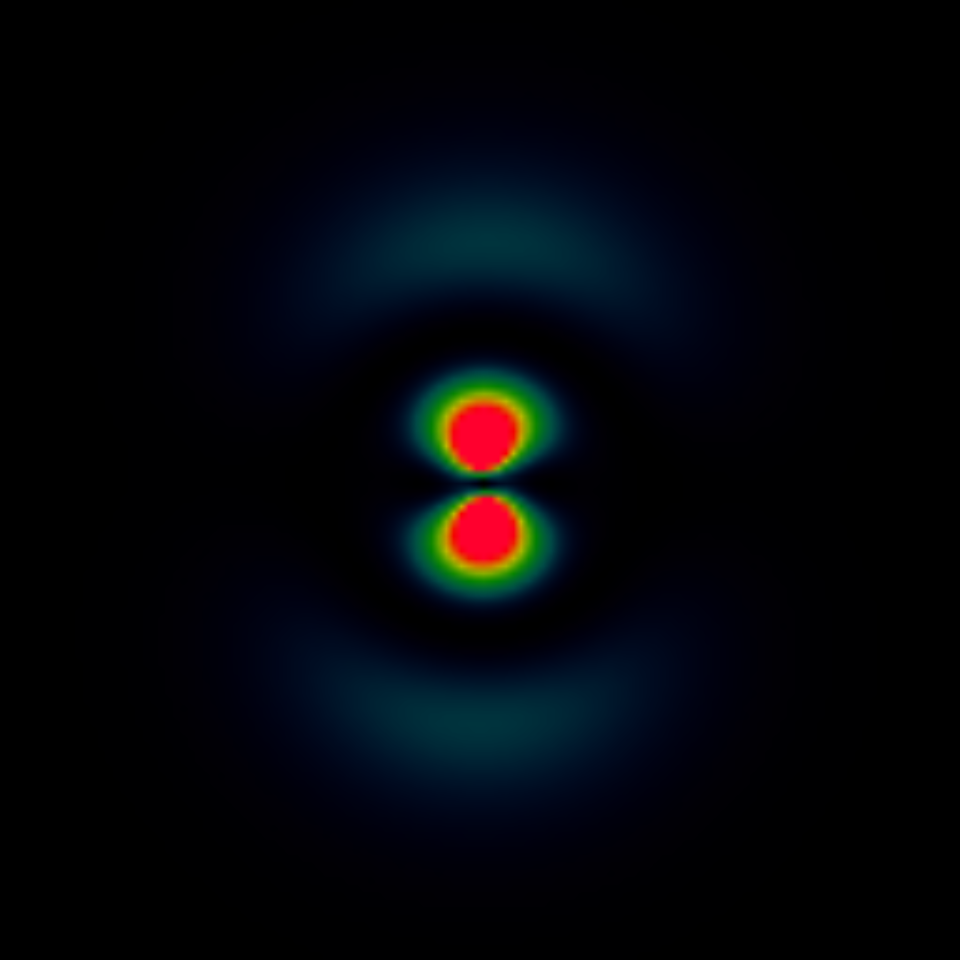
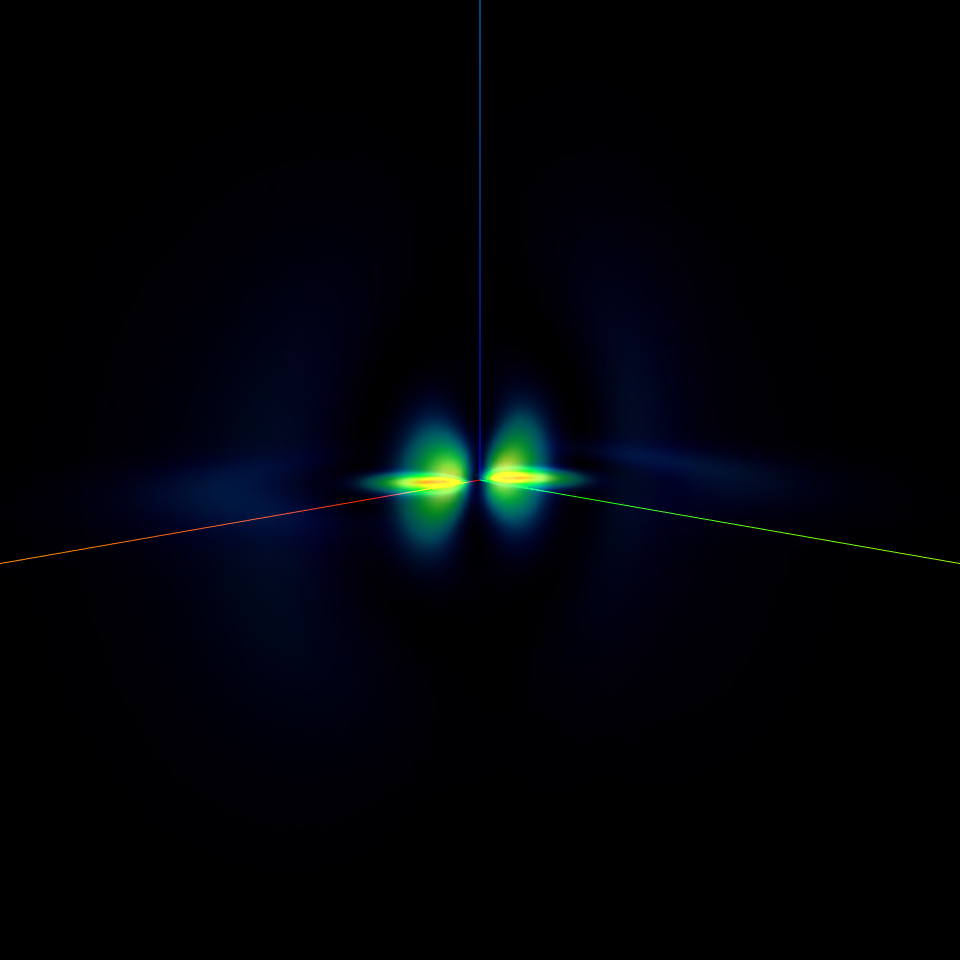
| $3p$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
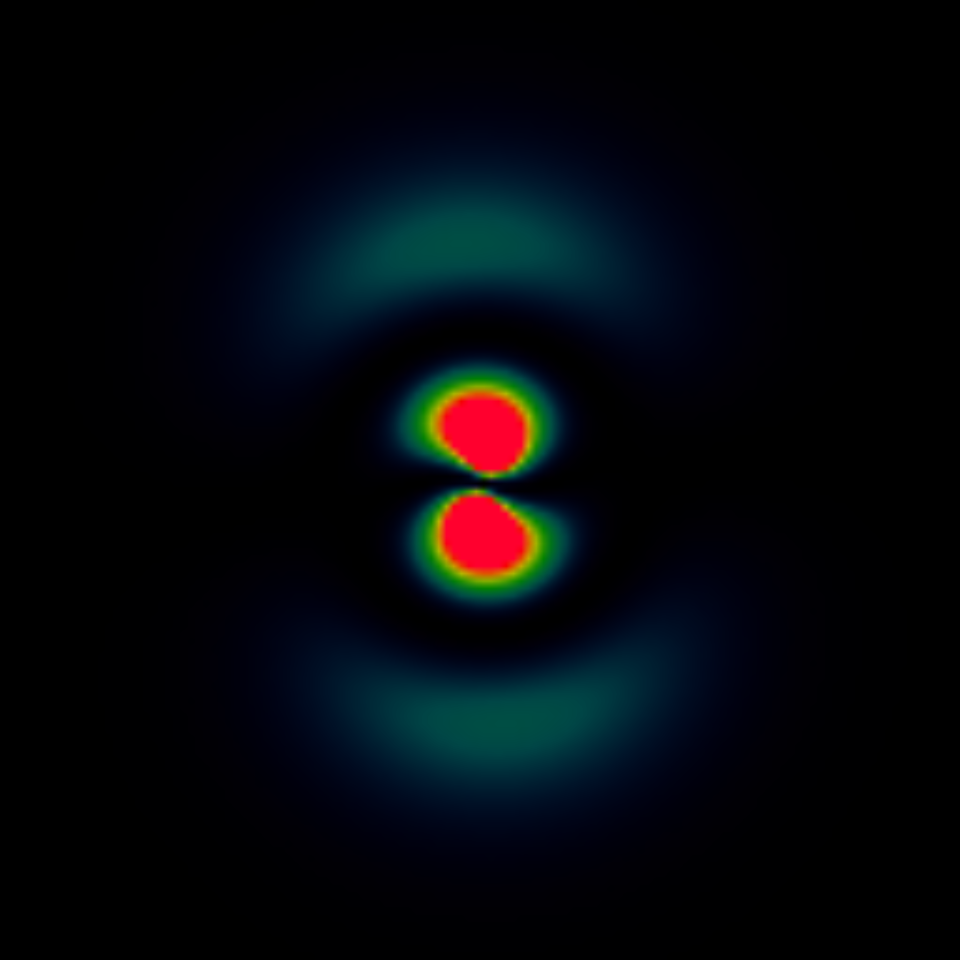
| $3p$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
| $3p$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
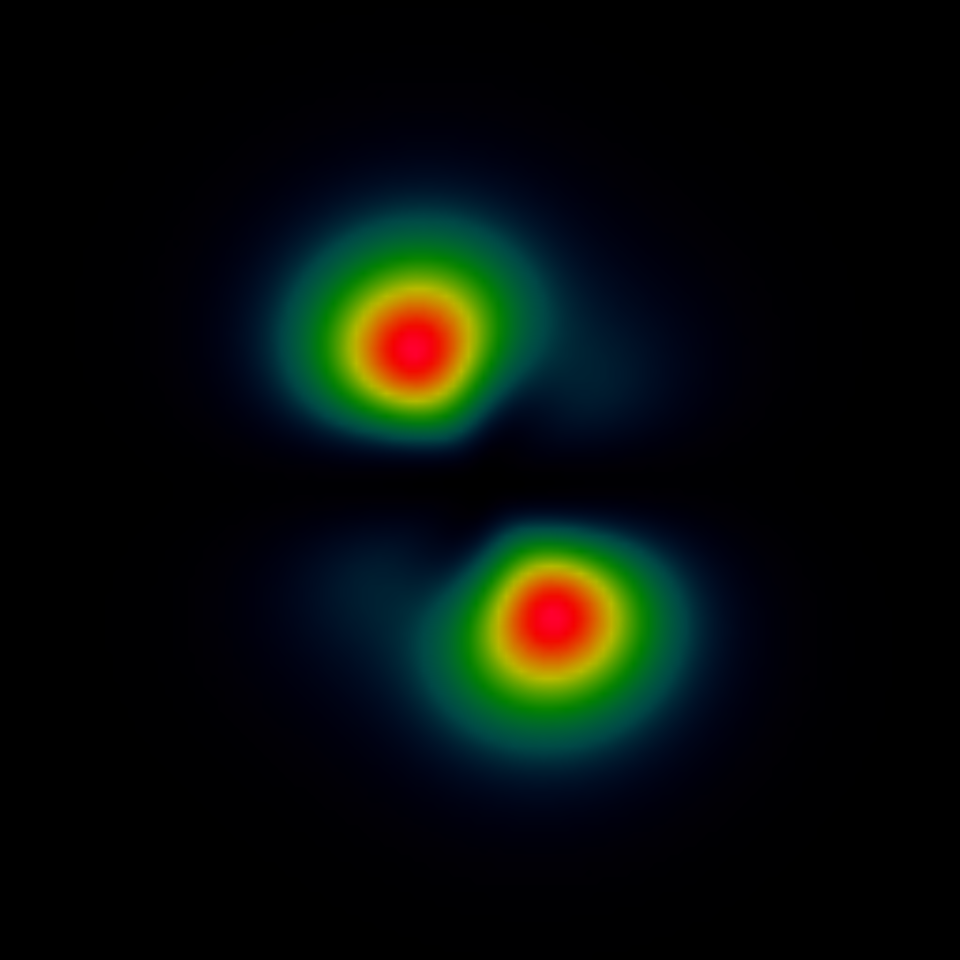
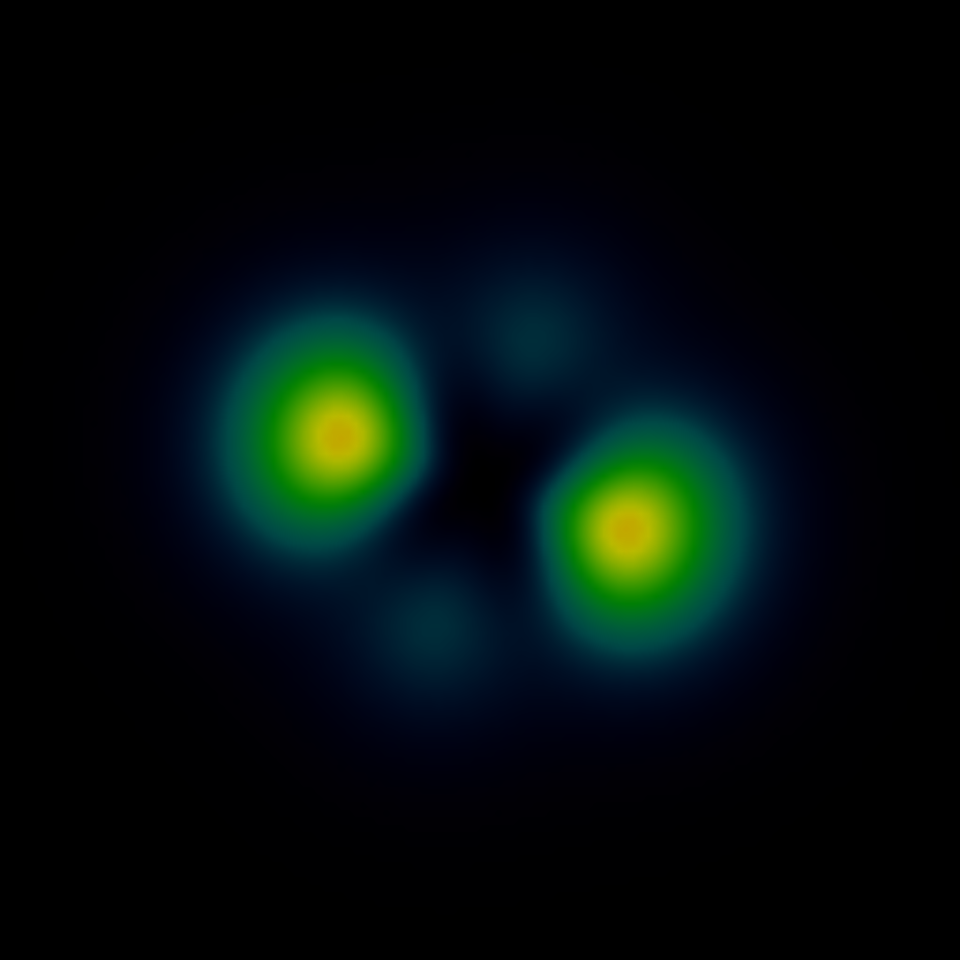
| $3d$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
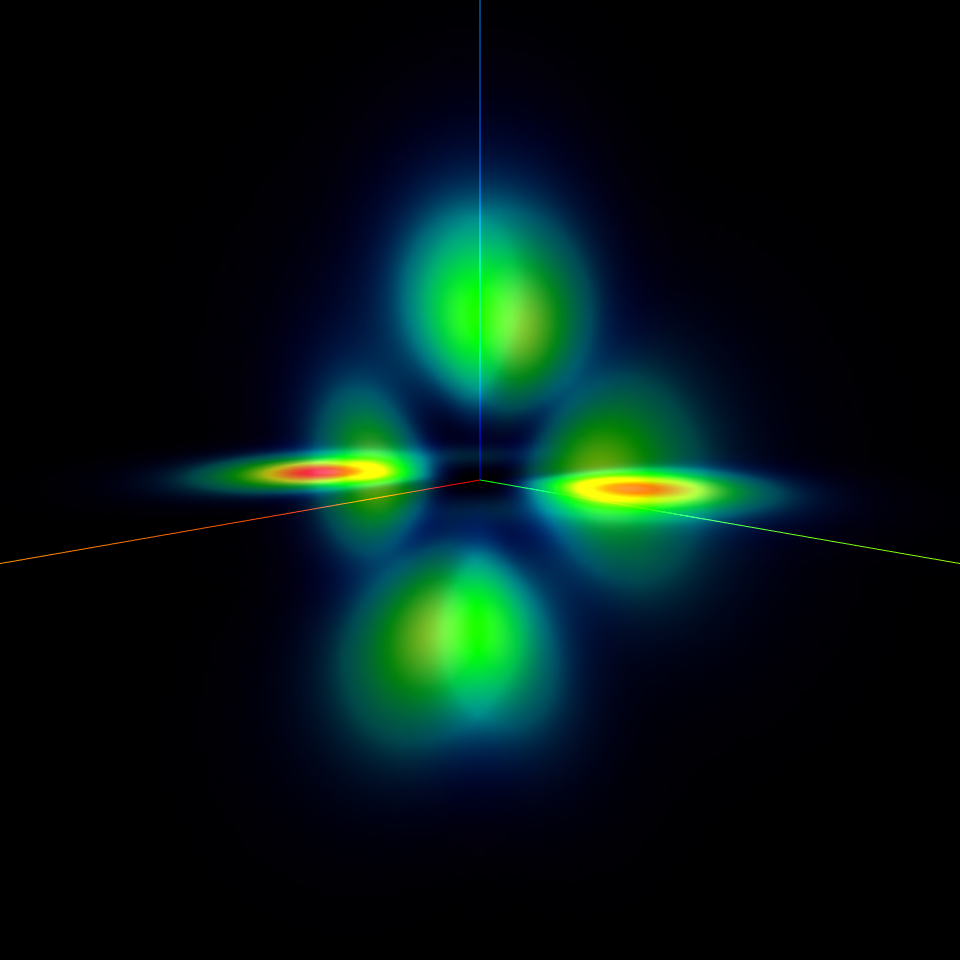
| $3d$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
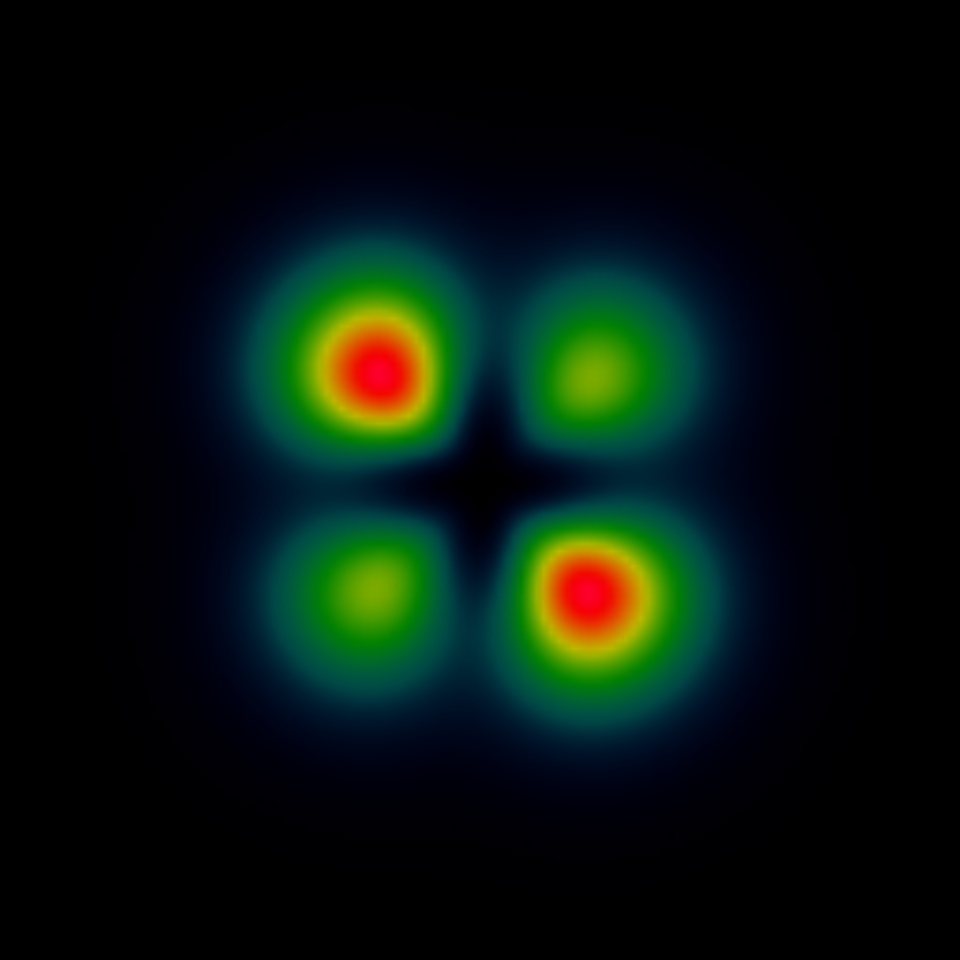
| $3d$ | - | - | - |  |
 |
 |
|
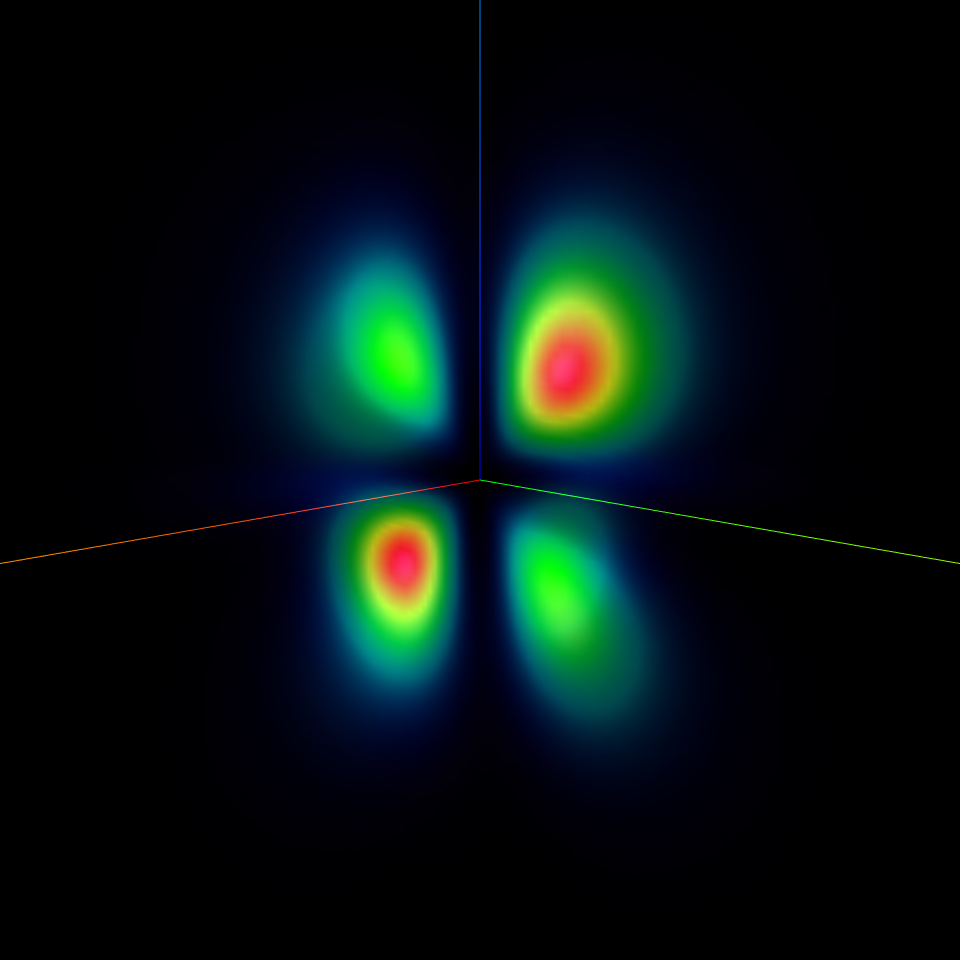
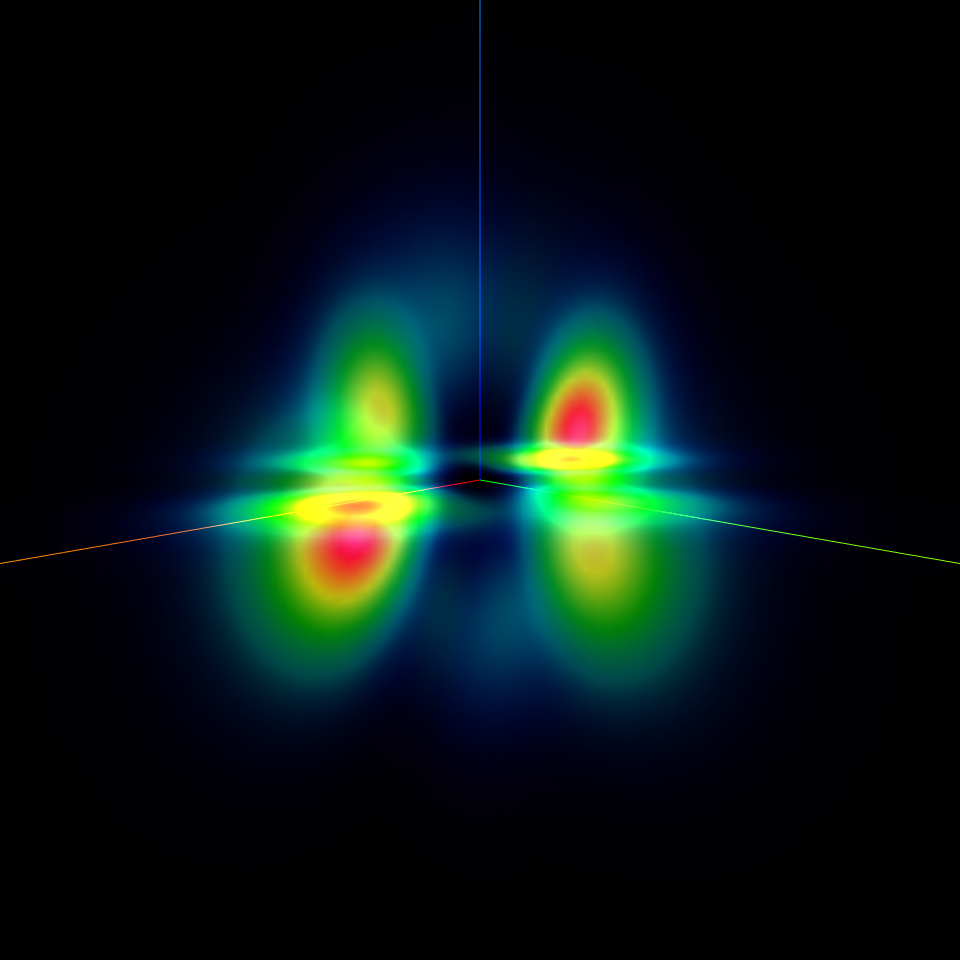
$L=40[a_B]$ |
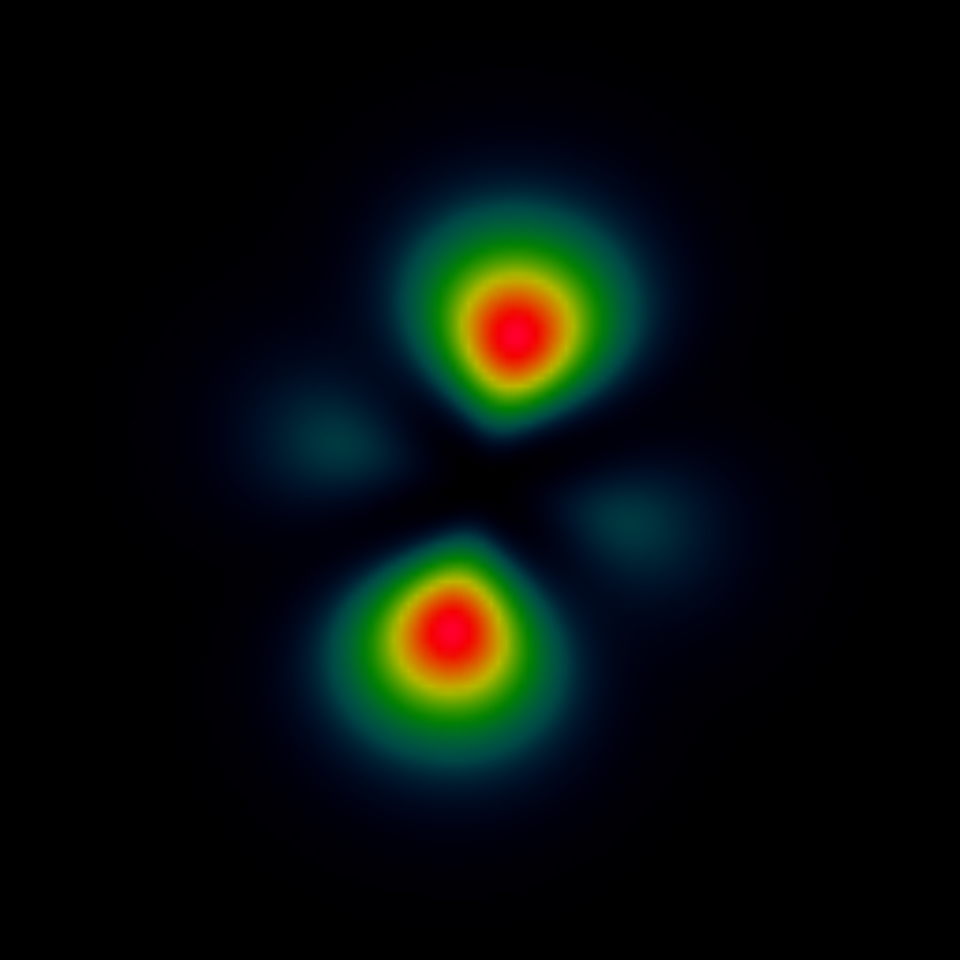
| $3d$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
| $3d$ | - | - | - |  |
 |
 |
|
$L=40[a_B]$ |
ちなみにヘリウム原子の場合、直交関数系は $(1,0,0)$ を必ず含むよ。なぜならば、$(1,0,0)$ から次に低い $(2,0,0)$ とした場合の固有エネルギーは約 $-20[{\rm eV}]$ で、イオン化エネルギーよりも高くなるために実質的には先に電離してしまうね。